CBSE
 Multiple Choice Questions
Multiple Choice Questionsસમગુણોત્તર
A.
સમગુણોત્તર
Tips: -
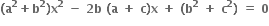 નાં બીજ સમાન છે.
નાં બીજ સમાન છે.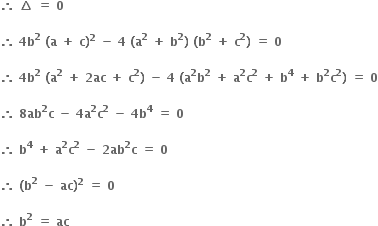
 હોય, તો
હોય, તો 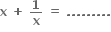
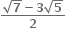
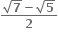
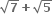

 નું એક બીજ ....... હોય.
નું એક બીજ ....... હોય.
3
8
4
2
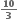 = log2y + logy2 તથા x ≠ y હોય તો x+y = ......
= log2y + logy2 તથા x ≠ y હોય તો x+y = ......

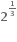
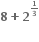
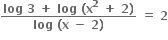 ના ....... ઉકેલ મળે.
ના ....... ઉકેલ મળે.
0
1
2
3
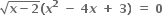 નાં વાસ્તવિક બીજ ...... હોય.
નાં વાસ્તવિક બીજ ...... હોય.
1,2,3
2, 3
1, 3
1, 2
 અને
અને 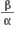 બીજ ધરાવતું દ્વિઘાત સમીકરણ ....... હોય.
બીજ ધરાવતું દ્વિઘાત સમીકરણ ....... હોય.0