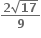CBSE
 Multiple Choice Questions
Multiple Choice QuestionsA.
a - b = 1B.
a = - bTips: -
ધારો કે સમીકરણો x2 - ax + b = 0 તથા x2 + bx - a = 0 નું સામાન્ય બીજ α છે.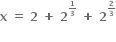 હોય તો x3 - 6x2 + 6x ની કિંમત ....... હોય.
હોય તો x3 - 6x2 + 6x ની કિંમત ....... હોય.
3
1
2
2/3
 માટે
માટે 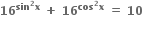 હોય તો x = .......... .
હોય તો x = .......... .
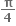

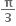

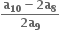
માત્ર એક જ કાલ્પનિક બીજ થાય.
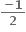
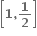
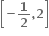
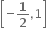
 હોય તો x ની શક્ય કિંમતો ..... હોય.
હોય તો x ની શક્ય કિંમતો ..... હોય.
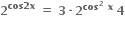 હોય તો x = ....... જ્યાં x ∈ [0,
હોય તો x = ....... જ્યાં x ∈ [0, ]
]

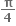
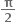
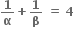 હોય તો |α -β| = ........ .
હોય તો |α -β| = ........ .