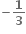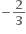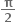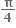CBSE
 Multiple Choice Questions
Multiple Choice QuestionsFTFT
TTFF
TFTT
FTTF
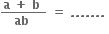
0
1
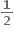
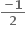
q



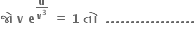
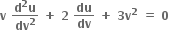
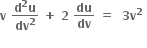
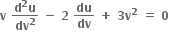
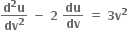
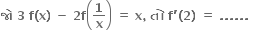
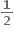
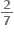
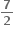
2
(b - c) f(a) + (c - b) f(b) > (c - a) f(c)
(b - a) f(c) + (c - b) f(a) > (c - a) f(b)
(b - a) f(c) + (c - b) f(a) < (c - a) f(b)
(b - c) f(a) + (c - b) f(b) > (c - a) f(c)
f(x) = sin x + cos x, 0 ≤ x ≤ 2 એ ...... અંતરાલમાં ચુસ્ત ઘટતું વિધેય છે.
એ ...... અંતરાલમાં ચુસ્ત ઘટતું વિધેય છે.
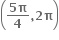
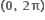
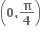
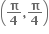
D.
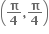
Tips: -
f(x) = sin x + cos x
f(x) = cos x - sin x
હવે, f'(x) = 0 ⇒ sin x = cos x. 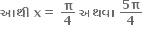
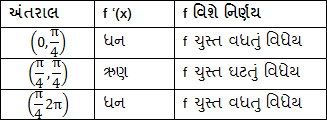
આમ, 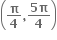 માં f ચુસ્ત ઘટતું વિધેય છે.
માં f ચુસ્ત ઘટતું વિધેય છે.
વિધેય f : (0, ∞) → (0, ∞) માટે,
(1) f(ab) = f(a) f(b) અને
(2)  f(x) = c, (જ્યાં ક # 0) પ્રકારનું છે. f(4) = ....
f(x) = c, (જ્યાં ક # 0) પ્રકારનું છે. f(4) = ....
1
2
3
4