CBSE
 Multiple Choice Questions
Multiple Choice Questions



x + 2y = 4
2x + y = 2
2x + y = 0
2x + y = 4
n મહત્તમ તથા n ન્યુનત્તમ મૂલ્ય મળે.
મહત્તમ કે ન્યુનતમ મુલ્ય ન મળે.
ફક્ત એક મહત્તમ મૂલ્ય મળે.
ફક્ત એક ન્યુનતમ મૂલ્ય મળે.
7
8
10
0
 તો
તો 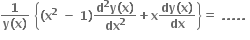
1
2
8
9
f'(1) = 0
2f(0) + f"(0) = 0
2f(0) + f"(0) = 2
f(2) = 0
A.
f'(1) = 0
C.
2f(0) + f"(0) = 2
Tips: -
ધારો કે f(x) = x3 + ax2 + bx - 2 કારણ કે f"'(0) = 6, f(0) = - 2
ધારો કે α, β, γ, ∈N એ f(x)= 0 નાં બીજ છે. આથી α β γ= - 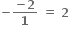
∴ α, β, γ માંથી ગને તે બેની કિંમત 1 હશે, જ્યારે ત્રિજાની કિંમત 2 હશે. ધારો કે α = 1, β = 1, γ = 2,
a = -(α + β + γ+ -4 = 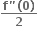 આથી f"(0) = - 8
આથી f"(0) = - 8
b = αβ + βγ + αγ = 5 = f'(0). આથી f'(0) = 5
∴ f(x) = x3 - 4x2 + 5x - 2
∴ 2f'(0) + f"(0) = 2
વળી, 1 એ f(x) = 0 નાં બે બીજ હોવાથી, f'(1) = 0
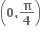 માં ઘટતું વિધેય છે.
માં ઘટતું વિધેય છે.
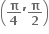 માં વધતું વિધેય છે.
માં વધતું વિધેય છે.
વિધેય g ને x = 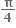 આગળ સ્થાનીય ન્યુનતમ મળે.
આગળ સ્થાનીય ન્યુનતમ મળે.
વિધેય g ને x = 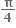 આગળ સ્થાનીય મહત્તમ મળે.
આગળ સ્થાનીય મહત્તમ મળે.
a < -3
-1 , a < 1
3 < a < 4
5 < a < 6
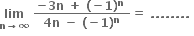
જો n અયુગ્મ સંખ્યા હોય તો 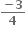
જો n યુગ્મ સંખ્યા હોય તો 
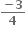
લક્ષનું અસ્તિત્વ નથી.
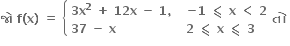
f ને x = 2 આગળ મહત્તમ કિંમત મળે.
f એ (10, 2) માં વધતું વિધેય છે.
f એ [-1, 3] પર સતત વિધેય છે.
f'(2)નું અસ્તિત્વ નથી.