CBSE
 Multiple Choice Questions
Multiple Choice Questions



x + 2y = 4
2x + y = 2
2x + y = 0
2x + y = 4
n મહત્તમ તથા n ન્યુનત્તમ મૂલ્ય મળે.
મહત્તમ કે ન્યુનતમ મુલ્ય ન મળે.
ફક્ત એક મહત્તમ મૂલ્ય મળે.
ફક્ત એક ન્યુનતમ મૂલ્ય મળે.
7
8
10
0
 તો
તો 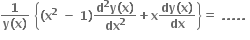
1
2
8
9
f'(1) = 0
2f(0) + f"(0) = 0
2f(0) + f"(0) = 2
f(2) = 0
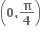 માં ઘટતું વિધેય છે.
માં ઘટતું વિધેય છે.
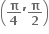 માં વધતું વિધેય છે.
માં વધતું વિધેય છે.
વિધેય g ને x = 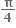 આગળ સ્થાનીય ન્યુનતમ મળે.
આગળ સ્થાનીય ન્યુનતમ મળે.
વિધેય g ને x = 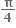 આગળ સ્થાનીય મહત્તમ મળે.
આગળ સ્થાનીય મહત્તમ મળે.
A.
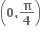 માં ઘટતું વિધેય છે.
માં ઘટતું વિધેય છે.
B.
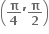 માં વધતું વિધેય છે.
માં વધતું વિધેય છે.
C.
વિધેય g ને x = 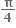 આગળ સ્થાનીય ન્યુનતમ મળે.
આગળ સ્થાનીય ન્યુનતમ મળે.
Tips: -
g'(x) = f"(sin x) cosx + f"(cos x) sin x
g'(x) = 0 ⇒ f'(sin x) cos x)f'(cos x) sin x
⇒f'(sin x) cos = f' 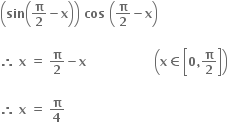
હવે, g"(x) = f"(sin x) cos2x + f"(cos x) sin2 x - {f'(sin x) sin x + f'(cos x) cos x} ...(1)
આપેલ છે કે f'(sin x) < 0, ∀ x ∈ R
∴ f' (cos x) < 0
(1) પરથી, g"(x) > 0, x ∈ R
∴ વિધેય g ને x = 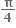 આગળ સ્થાનીય ન્યુનતમ મળે,
આગળ સ્થાનીય ન્યુનતમ મળે,
∴ g એ 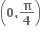 અંતરાલમાં ઘટતું વિધેય છે તથા
અંતરાલમાં ઘટતું વિધેય છે તથા 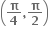 માં વધતું વિધેય છે.
માં વધતું વિધેય છે.
a < -3
-1 , a < 1
3 < a < 4
5 < a < 6
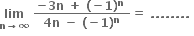
જો n અયુગ્મ સંખ્યા હોય તો 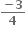
જો n યુગ્મ સંખ્યા હોય તો 
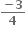
લક્ષનું અસ્તિત્વ નથી.
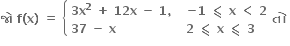
f ને x = 2 આગળ મહત્તમ કિંમત મળે.
f એ (10, 2) માં વધતું વિધેય છે.
f એ [-1, 3] પર સતત વિધેય છે.
f'(2)નું અસ્તિત્વ નથી.