CBSE
 Multiple Choice Questions
Multiple Choice Questions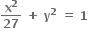 ને બિંદુ
ને બિંદુ  આગળ સ્પર્શક દોરેલ છે. આ સ્પર્શક દ્વારા કપાતા અંતઃખંડોનો સરવાળો ને એકઈ કિંમત માટે ન્યુનતમ થાય ?
આગળ સ્પર્શક દોરેલ છે. આ સ્પર્શક દ્વારા કપાતા અંતઃખંડોનો સરવાળો ને એકઈ કિંમત માટે ન્યુનતમ થાય ? 

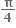

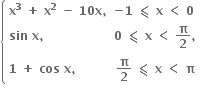 તો f ને
તો f ને
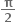 આગળ સ્થાનીય ન્યુનતમ મૂલ્ય મળે છે.
આગળ સ્થાનીય ન્યુનતમ મૂલ્ય મળે છે.
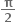 આગળ સ્થાનીય મહત્તમ મૂલ્ય મળે છે.
આગળ સ્થાનીય મહત્તમ મૂલ્ય મળે છે.
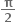 આગળ વૈશ્ચિક મહત્તમ મૂલ્ય મળે છે.
આગળ વૈશ્ચિક મહત્તમ મૂલ્ય મળે છે.
x= 0 આગળ વૈશ્વિક ન્યુનતમ મુલ્ય મળે છે.

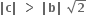
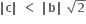
કોઈ સંબંધ ન હોય.
1
2
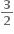
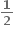
જો f વધતું વિધેય હોય તો h ઘટતું વિધેય થશે.
જો f ઘટતું વિધેય હોય તો h વધતું વિધેય થશે.
જો f વધતું વિધેય હોય તો h પણ વધતું વિધેય થશે.
h વિશે કઈ કહી શકાય નહિ.
1
2
3
4
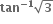
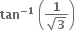
tan-1(-3)
tan-1 3
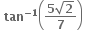
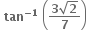
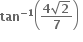
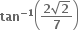
C.
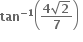
Tips: -
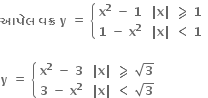
પ્રથમ બંને વક્રોનાં છેદબિંદુ શોધીએ.
|x2 - 1|= |x2 - 3|
x2 - 1 = ± 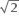 અને y = 1
અને y = 1
છેદબિંદુ A(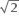 ,1) અને B(-
,1) અને B(-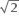 , 1) થશે.
, 1) થશે.
A(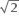 , 1) આગળ ખૂણાનું મપ શોધીએ.
, 1) આગળ ખૂણાનું મપ શોધીએ. 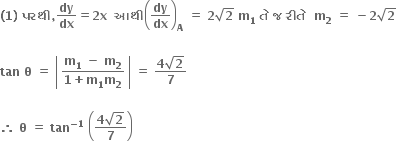
બિંદુ B આગળ પણ આ જ માપનો ખૂણો મળશે.
x = 0 આગળ વૈશ્વિક ન્યુનતમ મૂલ્ય મળે.
x = 0 આગળ મહત્તમ કે ન્યુનતમ વિશે કઈ કહી શકાય નહિ.
x = 0 આગળ વૈશ્વિક મહત્તમ મૂલ્ય મળે.
x = 0 આગળ વૈશ્વિક મહત્તમ મૂલ્ય કે વૈશ્વિક મૂલ્ય ન મળે.
tan-1 |t|
cot-1 |t|
tan-1 t2
cot-1 t2