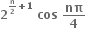CBSE
 Multiple Choice Questions
Multiple Choice Questions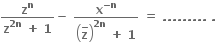
1
i
0
3
0
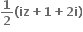
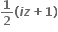
iz+1+i
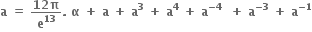 તથા
તથા  જેનાં બીજ હોય તેવું દ્વિઘાત સમીકરણ ......... છે.
જેનાં બીજ હોય તેવું દ્વિઘાત સમીકરણ ......... છે.x2 + x + 3 = 0
x2 - x + 2 = 0
x2 + x - 3 = 0
x3 - x - 3 = 0
x2 + x + 1 = 0
x2 + x - 1 = 0
x2 - x - 1 = 0
x2 - x + 1 = 0
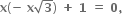 તો
તો  = ........... .
= ........... .
72
36
-72
0
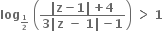 (જ્યાં
(જ્યાં 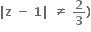 અસમતાનું પાલન કરતી સંકર સંખ્યાનો બિંદુગણ ......
અસમતાનું પાલન કરતી સંકર સંખ્યાનો બિંદુગણ ......વર્તુળનો બહારનો ભાગ.
વર્તુળ છે.
વર્તુળની અંદરનો ભાગ.
રેખા છે.
A.
વર્તુળનો બહારનો ભાગ.
Tips: -
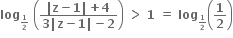
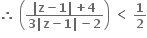 (loga એ a < 1 માટે ઘટતું વિધેય છે.)
(loga એ a < 1 માટે ઘટતું વિધેય છે.)
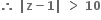 વર્તુળની બહારનો ભાગ છે.)
વર્તુળની બહારનો ભાગ છે.)
 એ ...... છે.
એ ...... છે. 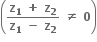
વાસ્તવિક અને ધન
શુદ્વ કાલ્પનિક સંખ્યા
વાસ્તવિક અને ઋણ
શૂન્ય સંખ્યા
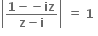 તો.......
તો.......
z એ શુદ્વ કાલ્પનિક સંખ્યા હોય.
P(z) એ બીજા ચરણમાં હોય.
P(z) એ ત્રીજા ચરણમાં હોય.
z એ વાસ્તવિક સંખ્યા હોય.
 હોય અને બાકીનાં બે બીજનો ગુણાકાર 13 + i હોય, તો b = ......... .
હોય અને બાકીનાં બે બીજનો ગુણાકાર 13 + i હોય, તો b = ......... .
51
15
6
30

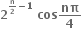
0