CBSE
 Multiple Choice Questions
Multiple Choice Questions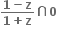
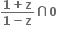
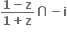

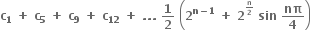

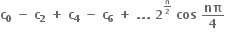
A.

Tips: -
(1 + x)n = c0 + c1 + c2x2 + c3x3 + ... (1)
(1) માં x = i મૂકતાં
(1+i)n = c0 + c1i - c2 - c3i + c4 + ... (2)
સમીકરણ (1) માં x = -i મૂકતાં
(1-i)n = c0 - c1i - c2 + c3i + c4 + ... (3)
(2) અને (3) નો સરવાળો કરતાં,
2 (c0 - c2 + c4 - c6 + ... ) = (1+i)n + (1-i)n
∴ c0 - c2 + c4 - c6 + ... = 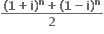
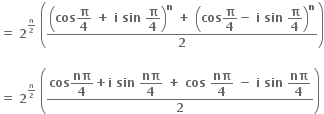
∴ c0 - c2 + c4 - c6 + ... =  (4)
(4)
તે જ રીતે (2) અને (3)ની બાદબાકી કરતાં,
2i (c1 - c3 + c5) = (1+i)n - (1-i)n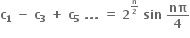 (5)
(5)
∴ આપણે જાણીએ છીએ કે c1 + c3 + c5 + ... = 2n-1 (6)
સમીકરણ (5) અને (6) નો સરવાળો કરતાં,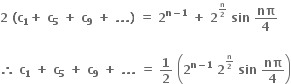
કાલ્પનિક અક્ષ પર હોય.
ઉપવલય પર હોય.
વર્તુળ પર હોય.
વાસ્તવિક અક્ષ પર હોય.
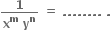

ઉપવલયની અંદરનો ભાગ
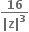 નું સમાધાન કરે તો |z|4 ની કિંમત ...... થાય.
નું સમાધાન કરે તો |z|4 ની કિંમત ...... થાય.1
2
3
4
1/2 ત્રિજ્યાવાળા વર્તુળ પર આવેલ છે.
 તો |z| ની ન્યુનતમ કિંમત ....... થાય.
તો |z| ની ન્યુનતમ કિંમત ....... થાય.




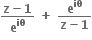 કાલ્પનિક ભાગ શુન્ય હોય, તથા
કાલ્પનિક ભાગ શુન્ય હોય, તથા 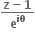 વાસ્તવિક ન હોય તો z એ
વાસ્તવિક ન હોય તો z એ
રેખા પર હોય.
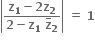 તથા |z2| ≠ 1. બિંદુ એ
તથા |z2| ≠ 1. બિંદુ એ
2 ત્રિજ્યાવાળા વર્તુળ પર હોય.