CBSE
 Multiple Choice Questions
Multiple Choice Questions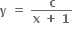 નો સ્પર્શક હોય, તો c = .......
નો સ્પર્શક હોય, તો c = .......
1
2
4
8
 માપનો ખુણો બનાવે છે તથા જે બિંદુના x - યામ અનુક્રમે 2 તથા 3 હોય તે બિંદુએ
માપનો ખુણો બનાવે છે તથા જે બિંદુના x - યામ અનુક્રમે 2 તથા 3 હોય તે બિંદુએ  તથા
તથા 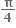 માપના ખૂણા બનાવે છે, તો
માપના ખૂણા બનાવે છે, તો 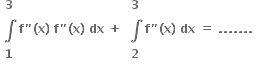
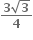
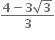

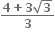
R

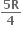

B.

Tips: -
જો નળાકાળની ત્રિજ્યા r અને ઊંચાઈ h હોય તો 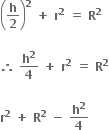
નળાકરનું ઘનફળ V =
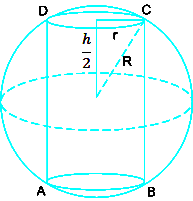
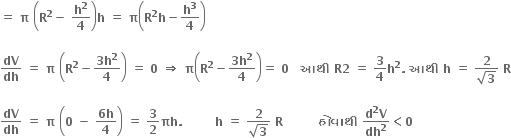
h =  R હોય ત્યારે નળાકારનું ઘનફળ મહત્તમ થશે.
R હોય ત્યારે નળાકારનું ઘનફળ મહત્તમ થશે.
G(x) એ વધતું વિધેય છે.
G(x) એ ઘટતું વિધેય છે.
G(x) એ એક-એક વિધેય છે.
G(x) એ વધતું કે ઘટતું વિધેય નથી.
 ] માં કેટલા ઉકેલ મળે ?
] માં કેટલા ઉકેલ મળે ?
1
2
3
0
0
2

(A) અથવા (c)
વિધેય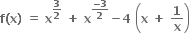 નું ન્યુનતમ મૂલ્ય ....... છે.
નું ન્યુનતમ મૂલ્ય ....... છે.
-10
10
0
ન મળે.
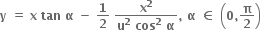 ના બિંદુ P આગળનો સ્પર્શક રેખા y = x + 5 ને સમાંતર છે. જો બિંદુ P નો y - યામ
ના બિંદુ P આગળનો સ્પર્શક રેખા y = x + 5 ને સમાંતર છે. જો બિંદુ P નો y - યામ  હોય તો α = ......
હોય તો α = ...... 
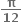

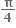
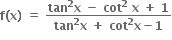 માટે નીચેનામાંથી કયા વિધાન સત્ય છે ?
માટે નીચેનામાંથી કયા વિધાન સત્ય છે ?
f(x)નું વૈશ્વિક ન્યુઅનતમ મૂલ્ય -1 છે.
f(x)નું વૈશ્વિક મહત્તમ મુલ્ય  છે.
છે.
f(x)ને વૈશ્વિક ન્યુનતમ મૂલ્ય ન મળે.
f(x)ને વૈશ્વિક મહતમ મૂલ્ય ન મળે.
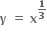 (1 - cos x) ને x = 0 આગળના સ્પર્શકનું સમીકરણ .... થશે.
(1 - cos x) ને x = 0 આગળના સ્પર્શકનું સમીકરણ .... થશે.
x = 0
x = 1
y = 0
y = 1