CBSE
 Multiple Choice Questions
Multiple Choice Questions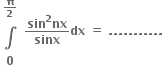
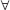 n ∈ N
n ∈ N
1
0
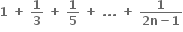
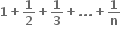
 વખત તો ગણિતીય અનુમાનના સિદ્વાંત પરથી કયું સત્ય છે?
વખત તો ગણિતીય અનુમાનના સિદ્વાંત પરથી કયું સત્ય છે?
 n ≥ 1
n ≥ 1
 n ≥ 1
n ≥ 1
 n ≥ 1
n ≥ 1
 n ≥ 1
n ≥ 1
C.
an < 4, n ≥ 1
n ≥ 1
Tips: -
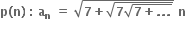 વખત
વખત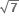 આથી a1 < 7 હોવાથી an > 7 સત્ય નથી.
આથી a1 < 7 હોવાથી an > 7 સત્ય નથી.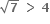 અને a1 =
અને a1 = 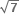 < 3 સત્ય છે.
< 3 સત્ય છે. 
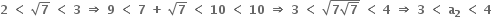
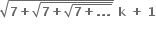 વખત
વખત 
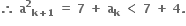 આથી
આથી 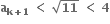 આથી
આથી 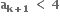
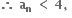
 n ∈ N
n ∈ NS(k) ⇒ S (k+1) સત્ય છે.
 અને I =
અને I = તો નીચેના પૈકી કયું દરેક n ≥ 1, n ∈ N માટે સત્ય છે ?
તો નીચેના પૈકી કયું દરેક n ≥ 1, n ∈ N માટે સત્ય છે ?