CBSE
 Multiple Choice Questions
Multiple Choice QuestionsC.
-2, -3Tips: -
log2 (x2 + 5x + 10) = 2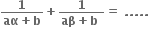

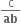
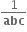
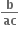
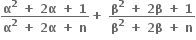 ની કિંમત ...... હોય.
ની કિંમત ...... હોય.
4
1
2
0
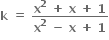 હોય તો k ∈ ....... .
હોય તો k ∈ ....... .
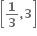
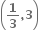
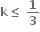
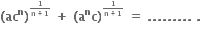
-ab
-b
-c
-a
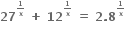 નાં વાસ્તવિક બીજોની સંખ્યા ....... છે.
નાં વાસ્તવિક બીજોની સંખ્યા ....... છે.
3
0
1
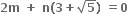
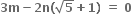
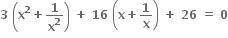 નો ઉકેલ ગણ ...... હોય.
નો ઉકેલ ગણ ...... હોય.
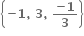
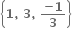
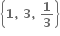
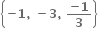
-6, -1
-6, 1
-1, 6
6, 1