CBSE
 Multiple Choice Questions
Multiple Choice Questions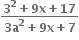 ની મહત્તમ કિંમત ........ હોય.
ની મહત્તમ કિંમત ........ હોય.
1
1/4
17/7
41
3
2
1
0
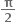 , હોય તથા સમીકરણ ax2 + bx + c = 0 (જ્યાં a ≠0)) નાં બીજ tan
, હોય તથા સમીકરણ ax2 + bx + c = 0 (જ્યાં a ≠0)) નાં બીજ tan  તથા
તથા  હોય, તો નીચેનામાંથી કયું સત્ય બને ?
હોય, તો નીચેનામાંથી કયું સત્ય બને ? C.
c = a + bTips: -
અહીં સમીકરણ ax2 + bx + c = 0 નાં બીજ tan અને tan
અને tan  છે.
છે. 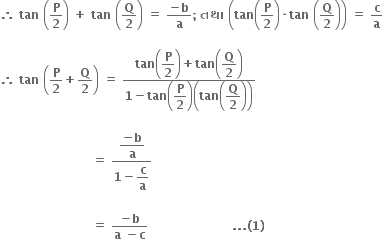



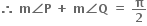 ... (2)
... (2)
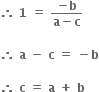
1 તથા 2 ની વચ્ચે