CBSE
 Multiple Choice Questions
Multiple Choice Questions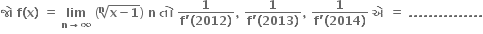
સ્વરિત શ્રેણિમાં હોય.
સમાંતર શ્રેણીમાં હોય.
સમગુણોત્તર શ્રેણીમાં હોય.
એક પણ નહિ.
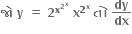 = ......
= ......

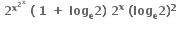

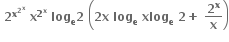
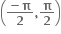 g(x) 2 tan-1 (ex)-
g(x) 2 tan-1 (ex)-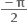 અને f એ g નું પ્રતિવિધેય હોય તો f'(0) = ........
અને f એ g નું પ્રતિવિધેય હોય તો f'(0) = ........ 4
3
2
1
વિધેય f : R → R માટે નીચે આપેલ પૈકી કયા વિધન સત્ય (T) કે મિથ્યા (F) છે ?
(1) જો |f(x) - f(y)|≤30 |x - y|, ∀x, y, ∈ R, તો f એ R પર સતત વિધેય છે.
(2) જો |f(x) - f(y)|≤30 |x - y|, ∀x, y, ∈ R, તો f એ R પર વિકલનીય વિધેય છે.
(3) જો |f(x) - f(y)|≤21 |x - y|2, ∀x, y, ∈ R, તો f એ R પર વિકલનીય વિધેય છે.
(4) જો |f(x) - f(y)|≤21 |x - y|2, ∀x, y, ∈ R, તો f એ અચળ વિધેય છે.
TFTF
FTTF
TFTT
TTTT
f એ વિકલનીય વિધેય છે તથા 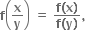 x#0, y#0, f(y) # 0 છે. જો f'(1) = 2 હોય તો f'(1) = 2 હોય તો f'(x) = .......
x#0, y#0, f(y) # 0 છે. જો f'(1) = 2 હોય તો f'(1) = 2 હોય તો f'(x) = .......
2x f(x)
2 f(x)
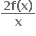

C.
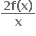
Tips: -
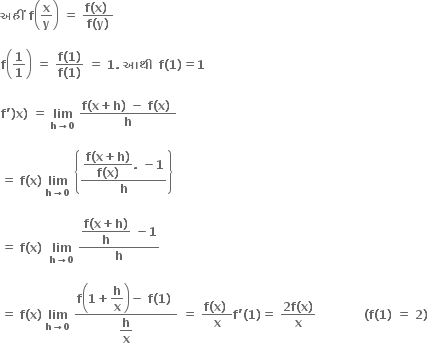
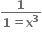 તો g(x) = .......
તો g(x) = ....... 1+[g(x)]3
1+ g(x)
g(x)
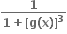
 = ........
= ........
4
3
2
1
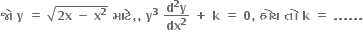
4
3
2
1
h(x) એ સતત વિધેય તેમજ વિકલનીય વિધેય છે.
h(x) એ સતત વિધેય છે અને ફક્ત x = 0 આગળ વિકલનીય છે.
h(x) એ અસતત વિધેય છે.
h(x) એ સતત વિધેય છે પરંતુ x = 0 આગળ વિકલનીય નથી.
0
3
5
8