Short Answer Type
Long Answer TypeUsing integration, find the area of the triangular region whose sides have the equations y = 2 x + 1, y = 3 x + 1 and x = 4.
The equations of the sides are
y = 2 x + 1 Â Â Â ...(1)
y = 3 x + 1 Â Â Â ...(2)
and x = 4. Â Â Â ...(3)
Subtracting (1) from (2), we get,
         
Putting x = 0 Â in (1), we get y = 0+1 = 1
  line (1) and (2) intersect in A(0, 1)
From (1) and (3), we get,
           
 line (1) and (3) intersect in B (4, 9)
From (2) and (3), we get,
x = 4, y = 12 + 1 = 13
∴  lines (2) and (3) intersect in C (4, 13)
∴ vertices of the triangle ABC are A(0, 1), B (4, 9), C (4, 13)
Required area = Area of ∆ ABC = Area of region AOMC - area of region AOMB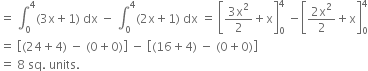
Using integration, find the area of the region bounded by the triangle whose vertices are (-1, 1), (0, 5) and (3, 2).
Short Answer TypeUsing integration, find the area of the region bounded by the triangle whose vertices are (1, 0), (2, 2) and (3, 1).
Long Answer TypeUsing integration find the area of region bounded by the triangle whose vertices are (-1, 0), (1, 3) and (3, 2).
.
Switch
