Short Answer Type
Long Answer TypeUsing integration, find the area of the triangular region whose sides have the equations y = 2 x + 1, y = 3 x + 1 and x = 4.
Using integration, find the area of the region bounded by the triangle whose vertices are (-1, 1), (0, 5) and (3, 2).
Let A(-1, 1), B(0, 5), C(3, 2) be the vertices of the given triangle.
The equation of AB is
or y = 4x + 5 ...(1)
The equation of BC is
or 
...(2)
The equation of CA is
or 
Required area = Area of ∆ ABC
= Area of region AMOB + area of region BONC - area of region AMNC
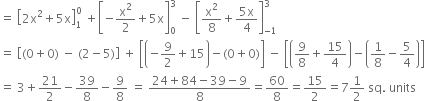
Short Answer TypeUsing integration, find the area of the region bounded by the triangle whose vertices are (1, 0), (2, 2) and (3, 1).
Long Answer TypeUsing integration find the area of region bounded by the triangle whose vertices are (-1, 0), (1, 3) and (3, 2).
.
Switch
