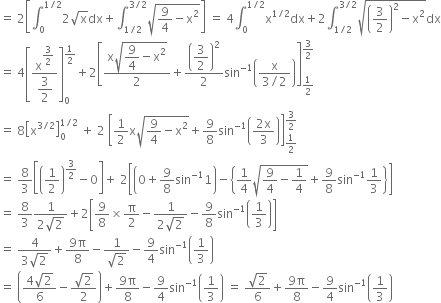Previous Year Papers

Download Solved Question Papers Free for Offline Practice and view Solutions Online.

Test Series

Take Zigya Full and Sectional Test Series. Time it out for real assessment and get your results instantly.

Test Yourself

Practice and master your preparation for a specific topic or chapter. Check you scores at the end of the test.

Application of Integrals

Name: Zigya - For The Curious Learner
Rating: 4.4 (740 reviews)

Short Answer Type

61.

Find the area of the region {(x, y): x² + y² ≤ 1 ≤ x + y}.

105 Views

Long Answer Type

62.

Find the area of the region bounded by the circle x² + y² = 1 and x + y = 1. Also draw a rough sketch.

151 Views

63.

Find the area of the region {(x, y): x² ≤ y ≤ |x|}.
Or
Find the area of the region bounded by the parabola y = x² and y = |x|.

178 Views

64. Draw the rough sketch and find the area of the region:
{(x, y) : y² ≤ 8 x, x² + x² ≤ 9}

149 Views

65. Find the area of the region {(x, y): y² ≤ 4 x, 4x² + 4 y² ≤ 9}

102 Views

66. Find the area lying above x-axis and included between the circle x² + y² = 8 x and inside of the parabola y² = 4 x.

975 Views

67. Calculate the area enclosed in the region:

open curly brackets left parenthesis straight x comma space straight y right parenthesis space semicolon space space straight x squared plus straight y squared space less or equal than space 1 space less than space straight x plus 1 half straight y close curly brackets

116 Views

68. Find the area of the circle x² + y² = 16 which is exterior to the parabola y² = 6x.

1016 Views

69. Find the area of the circle 4x² + 4y² = 9 which is interior to the parabola y²= 4 x.

Consider the equations
$straight y squared space equals space 4 straight x$ ...(1)
and $4 straight x squared plus 4 straight y squared equals 9$
i.e., $straight x squared plus straight y squared space equals space 9 over 4$ ...(2)
From (1) and (2), we get,
$straight x squared plus 4 straight x equals 9 over 4 space or space space 4 straight x squared plus 16 straight x minus 9 space equals space 0$

$therefore space space space straight x space equals space fraction numerator negative 16 plus-or-minus square root of 256 plus 144 end root over denominator 8 end fraction equals fraction numerator negative 16 plus-or-minus 20 over denominator 8 end fraction equals space 1 half comma space minus 9 over 2$

$therefore space space space space from space left parenthesis 1 right parenthesis comma space space space straight y squared space equals space 1 half$ $open square brackets because space space straight y squared equals negative 9 over 2 space does space not space give space real space points close square brackets$

$therefore space space space space straight y space equals space fraction numerator 1 over denominator square root of 2 end fraction comma space minus fraction numerator 1 over denominator square root of 2 end fraction$

$therefore space space space space curve space left parenthesis 1 right parenthesis space and space left parenthesis 2 right parenthesis space intersect space in space the space points$
$straight P open parentheses 1 half comma space fraction numerator 1 over denominator square root of 2 end fraction close parentheses space and space straight Q space equals space open parentheses 1 half comma space minus fraction numerator 1 over denominator square root of 2 end fraction close parentheses$

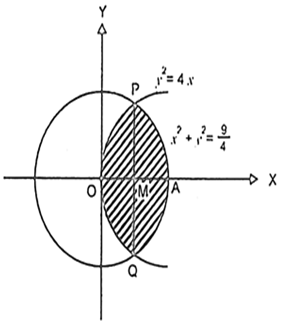
From P, draw $PM space perpendicular space straight x minus axis$
Here $OA space equals space 3 over 2$
Required area = Area of shaded region
= 2 (area of region OAPO) = 2 [area of region OMPO + area of region MAPM]

$equals space 2 open square brackets integral subscript 0 superscript 1 divided by 2 end superscript 2 square root of straight x dx plus integral subscript 1 divided by 2 end subscript superscript 3 divided by 2 end superscript square root of 9 over 4 minus straight x squared end root close square brackets space equals space 4 integral subscript 0 superscript 1 divided by 2 end superscript straight x to the power of 1 divided by 2 end exponent dx plus 2 integral subscript 1 divided by 2 end subscript superscript 3 divided by 2 end superscript square root of open parentheses 3 over 2 close parentheses squared minus straight x squared end root dx equals space 4 open square brackets fraction numerator straight x to the power of begin display style 3 over 2 end style end exponent over denominator begin display style 3 over 2 end style end fraction close square brackets subscript 0 superscript 1 half end superscript plus 2 open square brackets fraction numerator straight x square root of begin display style 9 over 4 minus straight x squared end style end root over denominator 2 end fraction plus open parentheses begin display style 3 over 2 end style close parentheses squared over 2 sin to the power of negative 1 end exponent open parentheses fraction numerator straight x over denominator 3 divided by 2 end fraction close parentheses close square brackets subscript 1 half end subscript superscript 3 over 2 end superscript equals space 8 open square brackets straight x to the power of 3 divided by 2 end exponent close square brackets subscript 0 superscript 1 divided by 2 end superscript space plus space 2 space open square brackets 1 half straight x square root of 9 over 4 minus straight x squared end root plus 9 over 8 sin to the power of negative 1 end exponent open parentheses fraction numerator 2 straight x over denominator 3 end fraction close parentheses close square brackets subscript 1 half end subscript superscript 3 over 2 end superscript equals space 8 over 3 open square brackets open parentheses 1 half close parentheses to the power of 3 over 2 end exponent minus 0 close square brackets plus space 2 open square brackets open parentheses 0 plus 9 over 8 sin to the power of negative 1 end exponent 1 close parentheses minus open curly brackets 1 fourth square root of 9 over 4 minus 1 fourth end root plus 9 over 8 sin to the power of negative 1 end exponent 1 third close curly brackets close square brackets equals space 8 over 3 fraction numerator 1 over denominator 2 square root of 2 end fraction plus 2 open square brackets 9 over 8 cross times straight pi over 2 minus fraction numerator 1 over denominator 2 square root of 2 end fraction minus 9 over 8 sin to the power of negative 1 end exponent open parentheses 1 third close parentheses close square brackets equals space fraction numerator 4 over denominator 3 square root of 2 end fraction plus fraction numerator 9 straight pi over denominator 8 end fraction minus fraction numerator 1 over denominator square root of 2 end fraction minus 9 over 4 sin to the power of negative 1 end exponent open parentheses 1 third close parentheses equals space open parentheses fraction numerator 4 square root of 2 over denominator 6 end fraction minus fraction numerator square root of 2 over denominator 2 end fraction close parentheses plus fraction numerator 9 straight pi over denominator 8 end fraction minus 9 over 4 sin to the power of negative 1 end exponent open parentheses 1 third close parentheses space equals space fraction numerator square root of 2 over denominator 6 end fraction plus fraction numerator 9 straight pi over denominator 8 end fraction minus 9 over 4 sin to the power of negative 1 end exponent open parentheses 1 third close parentheses$