Previous Year Papers

Download Solved Question Papers Free for Offline Practice and view Solutions Online.

Test Series

Take Zigya Full and Sectional Test Series. Time it out for real assessment and get your results instantly.

Test Yourself

Practice and master your preparation for a specific topic or chapter. Check you scores at the end of the test.

Application of Integrals

Name: Zigya - For The Curious Learner
Rating: 4.4 (740 reviews)

Multiple Choice Questions

81.

Area lying between the curves y² = 4x and y = 2x is

$2 over 3$
$1 third$
$1 fourth$
$1 fourth$

196 Views

82. Area bounded by the curve y = x³ the x-axis and the ordinates x - 2 and a = 1 is

-9
$fraction numerator negative 15 over denominator 4 end fraction$
$15 over 4$
$15 over 4$

110 Views

83. The area bounded by the curve y = x |x|, x-axis and the ordinates x = - 1 and x = 1 is given by

0
$1 third$
$2 over 3$
$2 over 3$

122 Views

84. The area of the circle x +y =16 exterior to the parabola y² = 6x is
$4 over 3 left parenthesis 4 straight pi minus square root of 3 right parenthesis$
$4 over 3 left parenthesis 4 straight pi plus square root of 3 right parenthesis$
$4 over 3 left parenthesis 8 straight pi minus square root of 3 right parenthesis$
$4 over 3 left parenthesis 8 straight pi minus square root of 3 right parenthesis$

4 over 3 left parenthesis 8 straight pi minus square root of 3 right parenthesis

The equation of circle is
$straight x squared plus straight y squared space equals space 16$ ...(1)
The equation of parabola is
   $straight y squared space equals space 6 straight x$ ...(2)
From (1) and (2),
   $straight x squared plus 6 straight x space equals space 16$
or $straight x squared plus 6 straight x minus 16 space equals space 0$
$therefore space space left parenthesis straight x plus 8 right parenthesis space left parenthesis straight x minus 2 right parenthesis space equals space 0$
$rightwards double arrow$ x = -8, 2
Rejecting x = -8 as parabola lies in 1st or 4th quadrant, we get x = 2
When x = 2,    $straight y equals square root of 12 space equals space 2 square root of 3$
$therefore$ (1) and (2) intersect in $straight P left parenthesis 2 comma space 2 square root of 3 right parenthesis$
Required Area = Area of the circle - area of circle interior to the parabola
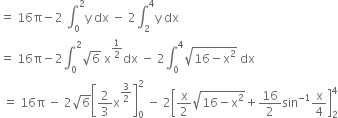
   $equals space 16 straight pi minus 2 space integral subscript 0 superscript 2 straight y space dx space minus space 2 integral subscript 2 superscript 4 straight y space dx equals space 16 straight pi minus 2 integral subscript 0 superscript 2 square root of 6 space straight x to the power of 1 half end exponent dx space minus space 2 integral subscript 0 superscript 4 square root of 16 minus straight x squared end root space dx space equals space 16 straight pi space minus space 2 square root of 6 open square brackets 2 over 3 straight x to the power of 3 over 2 end exponent close square brackets subscript 0 superscript 2 space minus space 2 open square brackets straight x over 2 square root of 16 minus straight x squared end root plus 16 over 2 sin to the power of negative 1 end exponent straight x over 4 close square brackets subscript 2 superscript 4$

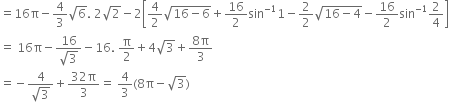
$equals 16 straight pi minus 4 over 3 square root of 6. space 2 square root of 2 minus 2 open square brackets 4 over 2 square root of 16 minus 6 end root plus 16 over 2 sin to the power of negative 1 end exponent 1 minus 2 over 2 square root of 16 minus 4 end root minus 16 over 2 sin to the power of negative 1 end exponent 2 over 4 close square brackets equals space 16 straight pi minus fraction numerator 16 over denominator square root of 3 end fraction minus 16. space straight pi over 2 plus 4 square root of 3 plus fraction numerator 8 straight pi over denominator 3 end fraction equals negative fraction numerator 4 over denominator square root of 3 end fraction plus fraction numerator 32 straight pi over denominator 3 end fraction equals space 4 over 3 left parenthesis 8 straight pi minus square root of 3 right parenthesis$

196 Views

85.

The area bounded by the x-axis, y = cosx and y = sin x when $0 less or equal than straight x less or equal than straight pi over 2$

$2 left parenthesis square root of 2 minus 1 right parenthesis$
$square root of 2 minus 1$
$square root of 2 plus 1$
$square root of 2 plus 1$

162 Views

Long Answer Type

86. Prove that the curves y² = 4x and x² = 4y divide the area of the square bounded by x = 0, x = 4, y = 4 and y = 0 into three equal parts.

640 Views

87.

Using the method of integration, find the area of the triangular region whose vertices are (2, -2), (4, 3) and (1, 2).

1133 Views

88.

Sketch the region bounded by the curves $straight y equals square root of 5 minus straight x squared end root space and space straight y space equals open vertical bar straight x minus 1 close vertical bar$ and find its area using integration.