Previous Year Papers

Download Solved Question Papers Free for Offline Practice and view Solutions Online.

Test Series

Take Zigya Full and Sectional Test Series. Time it out for real assessment and get your results instantly.

Test Yourself

Practice and master your preparation for a specific topic or chapter. Check you scores at the end of the test.

Circles

Short Answer Type

241. Two tangents TP and TQ are drawn from an external point T to a circle with centre O, as shown in figure, are inclined to each other at angle of 100°, then what is the value of ∠POQ?

2747 Views

242.

In figure PA and PB are tangents from P to the circle with centre O. R is a point on the circle. Prove that : PC + CR = PD + DR

1049 Views

243. The length of a tangent from a point A at a distance of 26 cm from the centre of the circles is 10 cm. What will be the radius of the circle?

125 Views

244. In figure, two concentric circles are of radii 5 cm and 3 cm. Find the length of the chord of the larger circle which touches the smaller cirles.

123 Views

245. In the figure given below, PA and PB are tangents to the circle drawn from an external point P. CD is a third tangent touching the circle at Q. If PB = 12 cm and CQ = 3 cm, what is the length of PC.

623 Views

246. The length of the tangent from a point A to circle, of radius 3 cm, is 4 cm. Find the distance of A from the centre of the circle.

103 Views

247. In figures, if TP and TQ are two tangents to a circle with centre O so that ∠POQ = 110°, then find ∠PTQ.

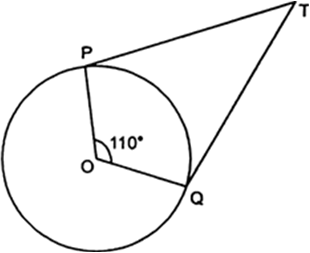
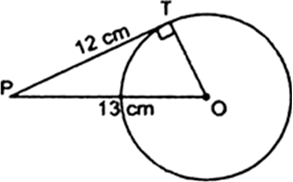
Since the tangent to a circle is perpendicular to the radius through the point of contact.
∴ ∠OPT = 90° = ∠OQT
In quad. OPTQ, we have
∠OPT + ∠PTQ + ∠OQT + ∠POQ = 360°
⇒ 90° + ∠PTQ + 90° + 110° = 360°
⇒ ∠PTQ = 70°

499 Views

248. In Fig. 10.28, ΔABC is circumscribing a circle. Find the length of BC.

95 Views

249. In the following figure, if AD, AE and BC are tangents to the circle at D, E and F respectively, then write 2AD in terms of AB, BC and CA.

196 Views

250. In figure, a point P is 13 cm away from the centre of the circle. The length of the tangent drawn from P to the circle is 12 cm. Find the radius of the circle.

328 Views