Short Answer Type
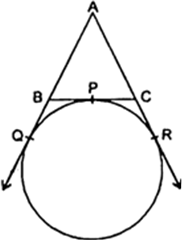
Short Answer TypeSince, the length of tangents drawn from an external point to a circle are equal.
So, BQ = BP
CP = CR and AQ = AR
Now, perimeter of ΔABC,
= AB + BC + AC
= AB + BP + PC + AC
= AB + BQ + CR + AC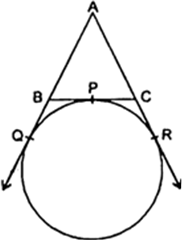
[∴ PB = BQ, PC = CR] = AQ + AR
= 2 AQ [∴ AQ = AR]![]()
 Long Answer Type
Long Answer Type Short Answer Type
Short Answer Type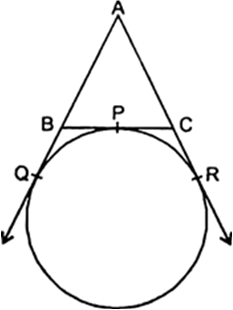
 Long Answer Type
Long Answer Type
 Short Answer Type
Short Answer TypeFrom a point P outside a circle, with centre O tangents PA and PB are drawn as shown in the figure, prove that
(i) ⇒AOP = ∠BOP
(ii) OP is the perpendicular bisector of AB.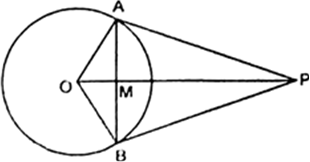
 Long Answer Type
Long Answer Type
 Short Answer Type
Short Answer Type