15. Draw an acute angled triangle ABC. Construct perpendicular bisectors of AB and BC intersecting each other at O. Measure OA, OB and OC. Are they equal? 

Steps of Construction

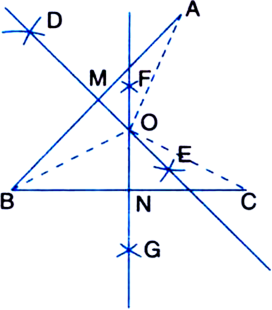
1. Draw an acute angled triangle ABC.
2. Taking B and A as centres and radius more than BA, draw arcs on both sides of the line segment BA (to intersect each other).

3. Let these arcs intersect each other at D and E. Join DE.

4. Let DE intersect BA at the point M. Then line DME is the required bisector of BA.

5. Again, taking B and C as centres and radius more than  BC, draw arcs on both sides of the line segment BC (to intersect each other).

6. Let these arcs intersect each other at F and G Join FG.
7. Let FG intersect BC at the point N. Then line FNG is the required bisector of BC.
8. Let the bisectors DME and FNG intersect each other at O.
By measure,
OA = OB = OC = 3.8 cm