Short Answer TypeFind the coordinates of a point A, where AB is the diameter of a circle whose centre is (2, – 3) and B is (1, 4).
Fig. 7.10.
Let the given point be A (x, y). Since C is the mid-point of AB.
∴ The co-ordinates of C are
But, the co-ordinates of C are (2, -3).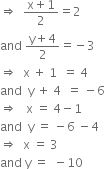
Hence, the co-ordinates of A are (3, -10).
If A and B are (– 2, – 2) and (2, – 4), respectively, find the coordinates of P such that 
and P lies on the line segment Ab.
Long Answer TypeFind the coordinates of the points which divide the line segment joining A(– 2, 2) and B(2, 8) into four equal parts.
Find the area of a rhombus if its vertices are (3, 0), (4, 5), (– 1, 4) and (– 2, – 1) taken in order.
Short Answer TypeIn each of the following find the value of ‘k’, for which the points are collinear
(7, –2), (5, 1), (3, k)
In each of the following find the value of ‘k’, for which the points are collinear
(8, 1), (k, – 4), (2, –5)
Find the area of the triangle formed by joining the mid-points of the sides of the triangle whose vertices are (0, –1), (2, 1) and (0, 3). Find the ratio of this area to the area of the given triangle.
Long Answer TypeFind the area of the quadrilateral whose vertices, taken in order, are (– 4, – 2), (– 3, – 5), (3, – 2) and (2, 3).
Switch
