 Short Answer Type
Short Answer Type Long Answer Type
Long Answer Type Short Answer Type
Short Answer Type Long Answer Type
Long Answer Type Short Answer Type
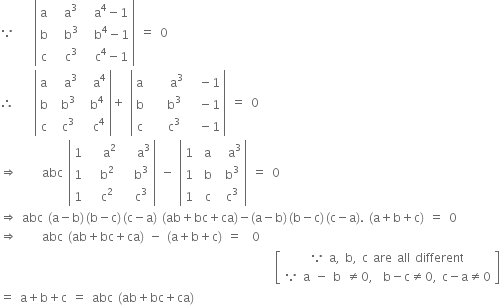
Short Answer TypeIf 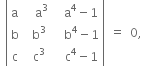
show that
a + b + c = abc(ab + bc + ca) where a , b , c are all different.
Let the three digit numbers A28,3B9 and 62C where A, B, and C are any integers between 0 and 9, be divisible by a fixed integer k. Show that the determinant
is divisible by k.
 Long Answer Type
Long Answer Type Multiple Choice Questions
Multiple Choice QuestionsLet A be a square matrix of order 3 × 3, then | kA | is equal to
