 Long Answer Type
Long Answer Type Short Answer Type
Short Answer Type Long Answer Type
Long Answer TypeEvaluate![]()
Let I = ![]()
Put ![]() ...(1)
...(1)
and ![]() ...(2)
...(2)
Squaring and adding (1) and (2), we get
![]()
![]()
Dividing (2) by (1), ![]()
![]()
![]()
![]()
![]()
![]()
![]()
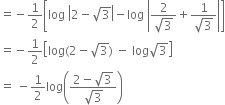
 Short Answer Type
Short Answer Type