Previous Year Papers

Download Solved Question Papers Free for Offline Practice and view Solutions Online.

Test Series

Take Zigya Full and Sectional Test Series. Time it out for real assessment and get your results instantly.

Test Yourself

Practice and master your preparation for a specific topic or chapter. Check you scores at the end of the test.

Quadrilaterals

Short Answer Type

31. In the given figure, PQRS is a parallelogram. B and D are mid-points of the sides QR and PS respectively. Show that ABCD is a parallelogram.

317 Views

32. In the given figure, PQRS is a parallelogram and ∠SPQ = 60°. If the bisectors of ∠P and ∠Q meet at A on RS, prove that A is the mid-point of RS.

181 Views

33.

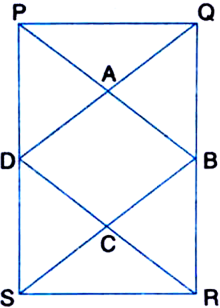
The angles A, B, C and D of a quadrilateral have measures in the ratio 2 : 4 : 5 : 7. Find the measures of these angles. What type of quadrilateral is it? Give reasons.

356 Views

34. If the diagonals of a quadrilateral bisect each other, prove that it is a parallelogram.

111 Views

35. In a parallelogram PQRS, if ∠QRS = 2x, ∠PQS = 4x and ∠PSQ = 4x, find the angles of the parallelogram.

126 Views

36.

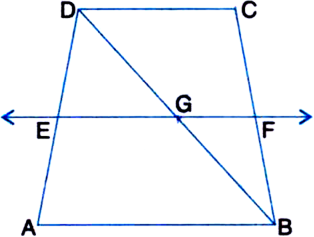
In the figure, ABCD is a parallelogram in which AB is produced to E so that AB = BE

(a) Prove that ED bisects BC
(b) If AD = 10 cm, find OB.

307 Views

Long Answer Type

37. ABCD is a quadrilateral in which P, Q, R and S are mid-points of the sides AB, BC, CD and DA (see figure). AC is a diagonal. Show that:

left parenthesis straight I right parenthesis space space SR parallel to space AC space and space SR space equals 1 half space AC

(ii) PQ = SR
(iii) PQRS is a parallelogram.

1502 Views

Short Answer Type

38. ABCD is a rhombus and P, Q, R and S are the mid-points of the sides AB, BC, CD and DA respectively. Show that the quadrilateral PQRS is a rectangle.

Given: ABCD is a rhombus. P, Q, R, S are the mid-points of AB, BC, CD, DA respectively. PQ, QR, RS and SP are joined.
To Prove: PQRS is a rectangle.
Construction: Join AC and BD.
Proof: In triangles RDS and PBQ,
DS = QB
| Halves of opposite sides of || gm ABCD which are equal
DR = PB
| Halves of opposite sides of || gm ABCD which are equal
∠SDR = ∠QBP
| Opposite ∠s of ||gm ABCD which are equal
∴ ∆RDS = ∆PBQ
| SAS Congruence Axiom
∴ SR = PQ | C.P.C.T.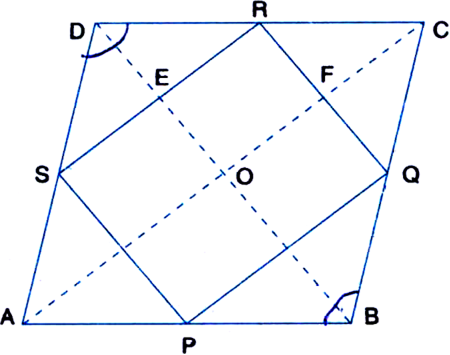

Given: ABCD is a rhombus. P, Q, R, S are the mid-points of AB, BC, CD
In triangles RCQ and PAS,
RC = AP
| Halves of opposite sides of || gm ABCD which are equal
CQ = AS
Halves of opposite sides of || gm ABCD which are equal
∠RCQ = ∠PAS
Opposite ∠s of || gm ABCD which are equal
∴ ∆RCQ ≅ ∆PAS
| SAS Congruence Axiom
∴ RQ = SP | C.P.C.T.
∴ In PQRS,
SR = PQ and RQ = SP
∴ PQRS is a parallelogram,
In ∆CDB,
∵ R and Q are the mid-points of DC and CB respectively.
∴ RQ || DB ⇒ RF || EO.
Similarly, RE || FO
∴ OFRE is a || gm
∴ ∠R = ∠EOF = 90°
| ∵ Opposite ∠s of a || gm are equal and diagonals of a rhombus intersect at 90°
Thus PQRS is a rectangle.

569 Views

39. ABCD is a rectangle and P, Q, R and S are mid-points of the sides AB, BC, CD and DA respectively. Show that the quadrilateral PQRS is a rhombus.

1569 Views

40. ABCD is a trapezium in which AB || DC, BD is a diagonal and E is the mid-point of AD. A line is drawn through E parallel to AB intersecting BC at F (see figure). Show that F is the midpoint of BC.

643 Views