Previous Year Papers

Download Solved Question Papers Free for Offline Practice and view Solutions Online.

Test Series

Take Zigya Full and Sectional Test Series. Time it out for real assessment and get your results instantly.

Test Yourself

Practice and master your preparation for a specific topic or chapter. Check you scores at the end of the test.

Real Numbers

Short Answer Type

81. Find the largest number which divides 245 and 1029 leaving remainder 5 in each case.

2073 Views

82. Find the largest number which divides 615 and 963 leaving remainder 6 in each case.

937 Views

83. What is the largest number that divides 626, 3127 and 15628 and leaves remainders of 1, 2 and 3 respectively.

1236 Views

Long Answer Type

84. Three sets of Physics, Chemistry and Biology books have to be stacked in such a way that all the books are stored topic-wise and the height of each stack is the same. The number of Physics books is 105, the number of Chemistry books is 140 and the number of Biology books is 175. Assuming that the books are of the same thickness, determine the number of stacks of Physics, Chemistry and Biology books.

166 Views

85. Using Euclid's division algorithm, find the HCF of 56, 96 and 404.

4892 Views

Short Answer Type

86. Find the LCIM and HCF of 6 and 20 by the Prime factorisation method.

158 Views

Long Answer Type

87. Find the LCM and HCF of 1296 and 2520 by the prime factorisation method.

473 Views

Short Answer Type

88.

Find the LCM and HCF of the following integers by applying the prime factorisation method :

12, 15 and 21

448 Views

89. Find the HCF of 96 and 404 by the prime factorisation method. Hence, Find their LCM.

Since 96 = 2 × 2 × 2 × 2 × 2 × 3

= 2^s × 3 and 404 = 2 × 2 × 101 = 2² × 101

Since 96 = 2 × 2 × 2 × 2 × 2 × 3
= 2s × 3 and 404 =

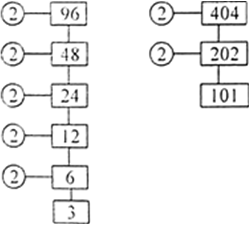
∴ LCM = Product of each prime factor with highest power

= 2^s × 3 × 101 = 9696

i.e., LCM (96, 404) = 9696

HCF Product of common prime factors with lowest powers

= 2² = 4

We know that,

HCF (a. b) × LCM (a,b) = a × b

⇒ HCF (96, 404) × LMC (96, 404) = 96 × 404

⇒ LCM (96, 404)

$<pre>uncaught exception: mkdir(): Permission denied (errno: 2) in /home/config_admin/public/felixventures.in/public/application/css/plugins/tiny_mce_wiris/integration/lib/com/wiris/util/sys/Store.class.php at line #56mkdir(): Permission denied in file: /home/config_admin/public/felixventures.in/public/application/css/plugins/tiny_mce_wiris/integration/lib/com/wiris/util/sys/Store.class.php line 56 #0 [internal function]: _hx_error_handler(2, 'mkdir(): Permis...', '/home/config_ad...', 56, Array) #1 /home/config_admin/public/felixventures.in/public/application/css/plugins/tiny_mce_wiris/integration/lib/com/wiris/util/sys/Store.class.php(56): mkdir('/home/config_ad...', 493) #2 /home/config_admin/public/felixventures.in/public/application/css/plugins/tiny_mce_wiris/integration/lib/com/wiris/plugin/impl/FolderTreeStorageAndCache.class.php(110): com_wiris_util_sys_Store->mkdirs() #3 /home/config_admin/public/felixventures.in/public/application/css/plugins/tiny_mce_wiris/integration/lib/com/wiris/plugin/impl/RenderImpl.class.php(231): com_wiris_plugin_impl_FolderTreeStorageAndCache->codeDigest('mml=<math xmlns...') #4 /home/config_admin/public/felixventures.in/public/application/css/plugins/tiny_mce_wiris/integration/lib/com/wiris/plugin/impl/TextServiceImpl.class.php(59): com_wiris_plugin_impl_RenderImpl->computeDigest(NULL, Array) #5 /home/config_admin/public/felixventures.in/public/application/css/plugins/tiny_mce_wiris/integration/service.php(19): com_wiris_plugin_impl_TextServiceImpl->service('mathml2accessib...', Array) #6 {main}</pre>$

630 Views

Long Answer Type

90.

Using prime factorization method, Find the LCM of

(i) 12, 15, 20, 27 (ii) 21, 28, 36, 45.

241 Views