Previous Year Papers

Download Solved Question Papers Free for Offline Practice and view Solutions Online.

Test Series

Take Zigya Full and Sectional Test Series. Time it out for real assessment and get your results instantly.

Test Yourself

Practice and master your preparation for a specific topic or chapter. Check you scores at the end of the test.

Statistics

Long Answer Type

291.

A survey regarding the heights (in cm) of 50 girls of class X of a school was conducted and the following data was obtained:

Height in cm	120 - 130	130 - 140	140 - 150	150 - 160	160 - 170	Total
Number of girls	2	8	12	20	8	50

1558 Views

292.

Find mean, median and mode of the following data:

Classes	Frequency
0-20	6
20-40	8
40-60	10
60-80	12
80-100	6
100-120	5
120-140	3

Median space equals space straight l plus open parentheses fraction numerator begin display style straight N over 2 end style minus straight c. straight f. over denominator straight f end fraction close parentheses cross times straight h equals 60 plus fraction numerator 25 minus 24 over denominator 12 end fraction cross times 20 equals 60 plus 20 over 12 equals space 60 plus 5 over 3 equals 60 plus 1.67 equals 61.67

642 Views

293.

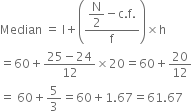
Find mean, median and mode of the following data:

Classes	Frequency
0 - 50	2
50 - 100	3
100 - 150	5
150 - 200	6
200 - 250	5
250 - 300	3
300 - 350	1

977 Views

294.
The following table gives the daily income of 50 workers of a factory :

Daily income (in Rs.)

No. of Workers

100-120

12

120-140

14

140-160

8

160-180

6

180-200

10

Find the Mean, Mode and Median of the above data.

C.I.	x_i	f_i	f_iix_i	c.f
100-120	110	12	1320	12
120-140	130	14	1820	26
140-160	150	8	1200	34
160-180	170	6	1020	40
180-200	190	10	1900	50
	Σf_i = 50		Σf_ix_i = 7260

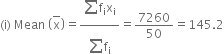
left parenthesis straight i right parenthesis space Mean space open parentheses top enclose straight x close parentheses equals fraction numerator begin display style sum from blank to blank of end style straight f subscript straight i straight x subscript straight i over denominator begin display style sum from blank to blank of end style straight f subscript straight i end fraction equals 7260 over 50 equals 145.2

(ii) Here the max. class frequency is 14, and the class corresponding to this frequency is 120-140.

therefore

Model class is 120 - 140,

therefore space space straight l space equals space 120 comma space

Class size h = 20

f₁= freq. of the model class = 14
f₀ = 12, f₂= 8

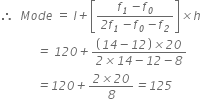
therefore space space M o d e space equals space l italic plus open square brackets fraction numerator f subscript italic 1 italic minus f subscript italic 0 over denominator italic 2 f subscript italic 1 italic minus f subscript italic 0 italic minus f subscript italic 2 end fraction close square brackets italic cross times h italic space italic space italic space italic space italic space italic space italic space italic space italic space italic space italic space italic space italic equals italic space italic 120 italic plus fraction numerator open parentheses italic 14 italic minus italic 12 close parentheses italic cross times italic 20 over denominator italic 2 italic cross times italic 14 italic minus italic 12 italic minus italic 8 end fraction italic space italic space italic space italic space italic space italic space italic space italic space italic space italic space italic space italic space italic equals italic 120 italic plus fraction numerator italic 2 italic cross times italic 20 over denominator italic 8 end fraction italic equals italic 125 italic space

left parenthesis iii right parenthesis space Here space straight n over 2 equals 25

which lies in the class 120 - 140

therefore space

l = 120. c.f. = 12, f = 14, h = 20

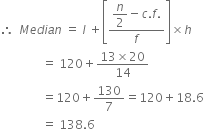
therefore space thin space M e d i a n space equals space l space plus open square brackets fraction numerator begin display style n over 2 end style minus c. f. over denominator f end fraction close square brackets cross times h space space space space space space space space space space space space space space equals space 120 plus fraction numerator 13 cross times 20 over denominator 14 end fraction space space space space space space space space space space space space space space equals 120 plus 130 over 7 equals 120 plus 18.6 space space space space space space space space space space space space space space equals space 138.6

Hence, mean = 145.2
mode = 125
median = 138.6

1455 Views

Short Answer Type

295.

Find the mode, median and mean for the following data :

Marks Obtained	Number of Students
25-35	7
35-45	31
45-55	33
55-65	17
65-75	11
75-85	1

197 Views

Long Answer Type

296.

The table given below shows the frequency distribution of the scores obtained by 200 candidates in a BCA examination.

Score	No. of candidates
200-250	30
250-300	15
300-350	45
350-400	20
400-450	25
450-500	40
500-550	10
550-600	15

Draw cumulative frequency curves by using (i) 'less than series', (ii) 'more than series'.

409 Views

297.

Draw both types of cumulative freqneucy curve on the same graph paper and then determine the median.

Marks obtained	No. of students
50-60	4
60-70	8
70-80	12
80-90	6
90-100	6

190 Views

298.

Draw 'less than' and 'more than' ogive curve from the following and indicate the value of median.

Marks	No. of students (f)
0-5	7
5-10	10
10-15	20
15-20	13
20-25	12
25-30	10
30-35	14
35-40	9

2485 Views

299. The following table gives the daily income of 50 workers of a factory. Draw both types ("less than type" and "greater than type") ogives and determine the median of the data.

Daily Income (in Rs.)	Number of Workers
100-120	12
120-140	14
140-160	8
160-180	6
180-200	10

1338 Views

300.

During the medical check-up of 35 students of a class their weights were recorded as follows:

Weight (in kg.)	No. of Students
38—40	3
40—42	2
42—44	4
44—46	5
46—48	14
48—50	4
50—52	3

Draw a less than type and a more than type ogive from the given data. Hence obtain the median weight from the graph.

782 Views

Daily income (in Rs.)	No. of Workers
100-120	12
120-140	14
140-160	8
160-180	6
180-200	10

Previous Year Papers

Test Series

Test Yourself

Long Answer Type

294. The following table gives the daily income of 50 workers of a factory : Daily income (in Rs.) No. of Workers 100-120 12 120-140 14 140-160 8 160-180 6 180-200 10 Find the Mean, Mode and Median of the above data.

Short Answer Type

Long Answer Type

294.
The following table gives the daily income of 50 workers of a factory :

Daily income (in Rs.)

No. of Workers

100-120

12

120-140

14

140-160

8

160-180

6

180-200

10

Find the Mean, Mode and Median of the above data.