Previous Year Papers

Download Solved Question Papers Free for Offline Practice and view Solutions Online.

Test Series

Take Zigya Full and Sectional Test Series. Time it out for real assessment and get your results instantly.

Test Yourself

Practice and master your preparation for a specific topic or chapter. Check you scores at the end of the test.

Surface Areas and Volumes

Short Answer Type

531. A hemispherical tank of radius 1.75 m is full of water it is connected with a pipe which empties it at the rate of 7 litres per second. How much time will it take to empty the tank completely.

505 Views

532. Four right circular cylindrical vessels each having diameter 21 cm. and height 38 cm. are full of icecream. The icecream is to be filled in cones of height 12 cm and diameter 7cm having hemispherical shape at the top. Find the total number of such cones which can be filled with icecream.

305 Views

Long Answer Type

533. A wooden article was made by scooping out a hemisphere from each end of a solid cylinder. If the height of the cylinder is 20 cm and radius of the base is 3.5 cm, find the total surface area of the article.

1148 Views

534.

The decorative block shown in fig. is made of two solids - a cube and a hemisphere. The base of the block is a cube with edge 5 cm, and the hemisphere fixed on the top has a diameter of 4.2 cm. Find the total surface area of the block.

673 Views

Short Answer Type

535. Mayank made a bird-bath for his garden in the shape of a cylinder with a hemispherical depression at one end. The height of the cylinder is 1.45 m and its radius is 30 cm. Find the total surface area of the birth - bath.

open parentheses bold pi bold space bold equals bold space bold 22 over bold 7 close parentheses bold space bold space

400 Views

536. A rocket is in the form of a circular cylinder closed at the lower end and a cone of the same radius is attached to the top The radius of the cylinder is 2.5 m, its height is 21 m and the slant height of the cone is 8 m. Calculate the total surface area of the rocket.

706 Views

Long Answer Type

537. A toy is in the form of a cone mounted on a hemisphere of common base radius 7cm. The total height of the toy is 31 cm. Find the total surface area of the toy.

384 Views

538. The interior of building is in the form of a right circular cylinder of radius 7 m and height 6 m, surmounted by a right circular cone of same radius and of vertical angle 60°. Find the cost of painting the building from inside at the rate of Rs. 30/m².

3277 Views

539. A solid is composed of a cylinder with hemispherical ends. If the whole length of the solid is 100 cm and the diameter of the hemispherical ends is 28 cm, find the cost of polishing the surface of the solid at the rate of 5 paise per sq. cm.

1223 Views

Short Answer Type

540. A solid toy is in the form of a hemisphere surmounted by a right circular cone. The height of the cone is 2 cm and the diameter of the base is 4 cm. Determine the volume of the toy. If a right circular cylinder circumscribes the toy.
Find the difference of the volumes of the cylinder and the toy.
(Use π = 3.14).

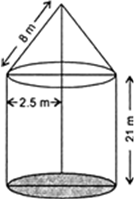
Let r cm be the radius and h cm be the height of a cone then.

$straight r space equals space 4 over 2 equals 2 space cm space and space straight h space equals space 2 space cm$

Let r₁ cm be the radius and h₁ cm be the height of hemisphere then,
r₁ = 2 cm, h₁ = 2 cm (∵ h₁= r₁]

Let r₂ cm be the radius and h₂ cm be the height of cylinder, then
r₂ = 2 cm
and h₂ = (2 + 2) cm = 4 cm
Now,
Volume of solid toy,
Volume of cone + Volume of hemisphere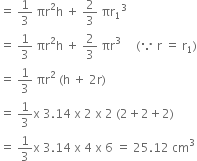
$equals space 1 third space πr squared straight h space plus space 2 over 3 space πr subscript 1 cubed equals space 1 third space πr squared straight h space plus space 2 over 3 space πr cubed space space space space space left parenthesis because space straight r space equals space straight r subscript 1 right parenthesis equals space 1 third space πr squared space left parenthesis straight h space plus space 2 straight r right parenthesis equals space 1 third straight x space 3.14 space straight x space 2 space straight x space 2 space left parenthesis 2 plus 2 plus 2 right parenthesis equals space 1 third straight x space 3.14 space straight x space 4 space straight x space 6 space equals space 25.12 space cm cubed$
Volume of cylinder,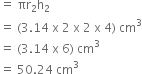
$equals space πr subscript 2 straight h subscript 2 equals space left parenthesis 3.14 space straight x space 2 space straight x space 2 space straight x space 4 right parenthesis space cm cubed equals space left parenthesis 3.14 space straight x space 6 right parenthesis space cm cubed equals space 50.24 space cm cubed$
Hence,
(i) Volume of toy = 25.12 cm
(ii) Difference of the volume of cylinder and the toy
= (50.24 – 25.12) cm³= 25.12 cm³.

1771 Views