Previous Year Papers

Download Solved Question Papers Free for Offline Practice and view Solutions Online.

Test Series

Take Zigya Full and Sectional Test Series. Time it out for real assessment and get your results instantly.

Test Yourself

Practice and master your preparation for a specific topic or chapter. Check you scores at the end of the test.

Triangles

Long Answer Type

221. In the given Fig, O is a point in the interior of a triangle ABC, OD ⊥ BC,OE ⊥ AC and OF ⊥ AB. Show that
(i) OA² + OB² + OC² - OD² - OE² - OF² = AF² + BD² + CE^2,(ii) AF² + BD² + CE² = AE² + CD² + BF².

1753 Views

Short Answer Type

222.

A ladder 10 m long reaches a window 8 m above the ground. Find the distance of the foot of the ladder from base of the wall.

139 Views

223. A guy wire attached to a vertical pole of height 18 m is 24 m long and has a stake attached to the other end. How far from the base of the pole should the stake be driven so that the wire will be taut?

136 Views

Long Answer Type

224. An aeroplane leaves an airport and flies due north at a speed of 1000 km per hour. At the same time, another aeroplane leaves the same airport and flies due west at a speed of 1200 km per hour. How far apart will be the two planes after

1 1 half hours ?

132 Views

225.

Two poles of heights 6 m and 11 m stand on a plane ground. If the distance between the feet of the poles is 12 m, find the distance between their tops.

156 Views

Short Answer Type

226. D and E are points on the sides CA and CB respectively of a triangle ABC right angled at C. Prove that AE² + BD² = AB² + DE².

1006 Views

Long Answer Type

227. The perpendicular from A on side BC of a ∆ABC intersects BC at D such that DB = 3 CD. Prove that 2AB² = 2AC² +BC².

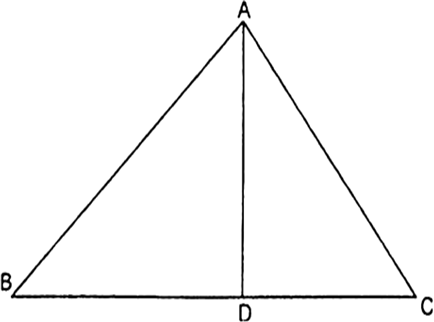
Given: A triangle ABC in which AD ⊥ BC and DB = 3CD.
To prove: 2AB² = 2AC² + BC²
Proof: ∵ DB = 3CD [given]
Now, BC = CD + DB
$rightwards double arrow$ BC = CD+ 3CD
$rightwards double arrow$ BC = 4CD
$rightwards double arrow$ $space CD equals 1 fourth BC$
Now, in right triangle ADB. we have
AB² = AD² + DB²
[Using Pythagoras Theorem]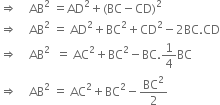
$rightwards double arrow space space space space AB squared space equals AD squared plus left parenthesis BC minus CD right parenthesis squared rightwards double arrow space space space space AB squared space equals space AD squared plus BC squared plus CD squared minus 2 BC. CD rightwards double arrow space space space space AB squared space space equals space AC squared plus BC squared minus BC.1 fourth BC rightwards double arrow space space space space AB squared space equals space AC squared plus BC squared minus BC squared over 2$

$rightwards double arrow space space AB squared space equals space fraction numerator 2 AC squared plus 2 BC squared minus BC squared over denominator 2 end fraction$

$rightwards double arrow$ $<pre>uncaught exception: mkdir(): Permission denied (errno: 2) in /home/config_admin/public/felixventures.in/public/application/css/plugins/tiny_mce_wiris/integration/lib/com/wiris/util/sys/Store.class.php at line #56mkdir(): Permission denied in file: /home/config_admin/public/felixventures.in/public/application/css/plugins/tiny_mce_wiris/integration/lib/com/wiris/util/sys/Store.class.php line 56 #0 [internal function]: _hx_error_handler(2, 'mkdir(): Permis...', '/home/config_ad...', 56, Array) #1 /home/config_admin/public/felixventures.in/public/application/css/plugins/tiny_mce_wiris/integration/lib/com/wiris/util/sys/Store.class.php(56): mkdir('/home/config_ad...', 493) #2 /home/config_admin/public/felixventures.in/public/application/css/plugins/tiny_mce_wiris/integration/lib/com/wiris/plugin/impl/FolderTreeStorageAndCache.class.php(110): com_wiris_util_sys_Store->mkdirs() #3 /home/config_admin/public/felixventures.in/public/application/css/plugins/tiny_mce_wiris/integration/lib/com/wiris/plugin/impl/RenderImpl.class.php(231): com_wiris_plugin_impl_FolderTreeStorageAndCache->codeDigest('mml=<math xmlns...') #4 /home/config_admin/public/felixventures.in/public/application/css/plugins/tiny_mce_wiris/integration/lib/com/wiris/plugin/impl/TextServiceImpl.class.php(59): com_wiris_plugin_impl_RenderImpl->computeDigest(NULL, Array) #5 /home/config_admin/public/felixventures.in/public/application/css/plugins/tiny_mce_wiris/integration/service.php(19): com_wiris_plugin_impl_TextServiceImpl->service('mathml2accessib...', Array) #6 {main}</pre>$
$rightwards double arrow$ $space 2 AB squared space equals space 2 AC squared plus BC squared.$