 Long Answer Type
Long Answer TypeIn the given Fig, two triangles ABC and DBC lie on the same side of base BC. P is a point on BC such that PQ || BA and PR || BD. Prove that: QR || AD. 
 Short Answer Type
Short Answer TypeIn the given fig, ST || QR, PS = 2 cm and SQ = 3 cm. What is the ratio of the area of ∆PQR to the area of ∆PST?
In the given Fig. PB and QA are perpendiculars to segment AB. If PO = 5 cm, QO = 7 cm and area ∆POB = 150 cm2 find the area of ∆QOA.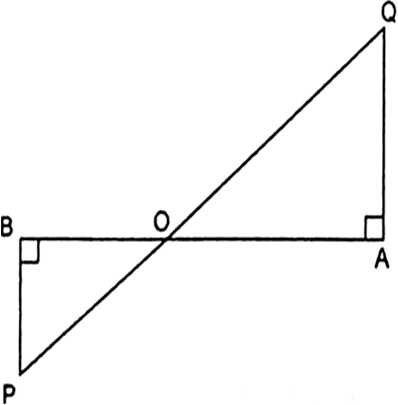
 Long Answer Type
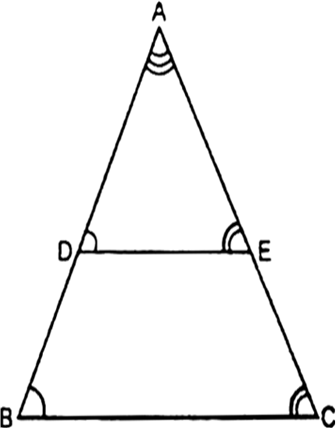
Long Answer TypeIn ∆BDE and ∆ABC
∠B = ∠B [Common]
∴ ∠1 = ∠2 [Correspondeing ∠S]
Now, ∆BDE ~ ∆ABC
∴ ![]()
We have, ![]()
![]()
![]()
![]()
![]()
![]()
![]()
![In ∆BDE and ∆ABC∠B = ∠B [Common]∴ ∠1 = ∠2 [](/application/zrc/images/qvar/MAEN10042245-8.png)
Putting (ii) in (i), we get
![]()
![]()
![]()
(Taking reciprocals of both side)
 Short Answer Type
Short Answer TypeIn the given fig, DE is parallel to BC and AD = 1 cm, BD = 2 cm. What is the ratio of the area of ∆ABC to the area of ∆ADE?
