Long Answer TypeLet 
Find a vector 
which is perpendicular to both 
and 
Here 
Let 
Since 
is perpendicular to 
and 3d2 – d3 = 0 ...(2)
Also, 
...(3)
Multiplying (1) by 3 and (2) by 1, we get,
3d1 – 3d2 = 0
3d2 – d3 = 0
Adding, 3d1 – d3 = 0 ...(4)
Subtracting (4) from (3), we get,
From (1), 
From (2), 
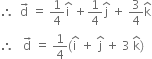
Short Answer TypeLong Answer TypeShort Answer TypeLet 
be three vectors of magnitude 5, 3, 1 respectively. If each one is perpendicular to the sum of other two vectors, prove that 
Long Answer TypeIf 
are mutually perpendicular vectors of equal magnitude, show that they are equally inclined to the vector 
Find a vector 
Switch
are 
are 
show that the angle 
between the vectors 
is given by
show that the angle between 
is 
are three mutually 
