 Long Answer Type
Long Answer TypeProve, using vectors, that the altitudes of a triangle are concurrent.
OR
Prove that the perpendicular from the vertices to the opposite sides of a triangle are concurrent.
Let ABC be given triangle and H be the point of intersection of altitudes AL and BM. Join CH and produce it to meet BA in N.
Take H as origin.
Let ![]() be the position vectors of A, B, C respectively.
be the position vectors of A, B, C respectively.![]()
Since ![]()

Again, ![]()
![]()
![]() ...(2)
...(2)
Adding (1) and (2), we get,
![]()
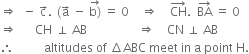
 Short Answer Type
Short Answer Type Long Answer Type
Long Answer Type Short Answer Type
Short Answer Type