 Long Answer Type
Long Answer Type Short Answer Type
Short Answer TypeLet ![]() be position vectors of A, B, C repsectively.
be position vectors of A, B, C repsectively.![]()
Now, ![]()
![]()
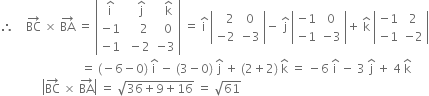
Area of ![]()
 Long Answer Type
Long Answer Type Short Answer Type
Short Answer TypeIf ![]() are the position vectors of the non-collinear points A, B, C respectively in space, show that
are the position vectors of the non-collinear points A, B, C respectively in space, show that ![]() is perpendicular to plane ABC.
is perpendicular to plane ABC.
 Long Answer Type
Long Answer Type Short Answer Type
Short Answer Type