 Multiple Choice Questions
Multiple Choice QuestionsThe area bounded by and x - axis is
sq unit
sq unit
D.
Required area
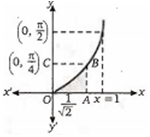
= Area of rectangle OABC - Area of curve OBCO
The figure shows a triangle AOB and the parabola y = x2. The ratio of the area of the triangle AOB to the area of the region AOB of the parabola y = x is equal to

The area of the plane region bounded by the curve x = y2 - 2 and the· line y = - x is (in square units)
Area bounded by the curve y = log (x - 2), x-axis and x = 4 is equal to
2log(2) + 1
log(2) - 1
log(2) + 1
2log(2) - 1
