 Multiple Choice Questions
Multiple Choice QuestionsThe area bounded by the parabola y2 = x, straight line y = 4 and y-axis is square units is
16/3 sq. unit
64/3 sq. unit
sq. unit
None of these
The area of the region bounded by the curves, y2 = 8x and y = x is
B.
Given curves,
y2 = 8x ...(i)
and y = x ...(ii)
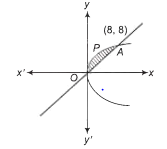
On solving Eqs. (i) and (ii), we get
x2 - 8x = 0
x(x - 8) = 0
The area of the region bounded by the lines y = 2x + 1, y = 3x + 1and x = 4 is
16 sq unit
8 sq unit
A closed figure S is bounded by the hyperbola x2 - y2 = a2 and the straight line x = a + h; (h > 0, a > 0). This closed figure is rotated about the X-axis. Then, the volume of the solid ofrevolution is
The centre of gravity (centre of mass) of a rod (of length L), whose linear mass density varies as the square of the distance from one end is at
The part of straight line y = x between x = 1 and x = 2 is revolved about X-axis, then the curved surface area of the solid thus generated is
None of these
The area of the portion of the circle x2 + y2 = 64 which is exterior to the parabola y2 = 12x, is
None of the above
For which of the followmg value of m, is the area of the region bounded by the curve y = x - x2 and the line y = mx equals to ?
- 4
- 2
2
- 4
