 Multiple Choice Questions
Multiple Choice QuestionsFor the Hyperbola  which of the following remains constant when α varies?
which of the following remains constant when α varies?
Eccentricity
Directrix
Abscissae of vertices
Abscissae of vertices
The equation of a tangent to the parabola y2 = 8x is y = x + 2. The point on this line from which the other tangent to the parabola is perpendicular to the given tangent is
(−1, 1)
(0, 2)
(2, 4)
(2, 4)
The locus of the vertices of the family of parabolas 
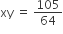
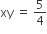
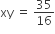
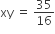
A.
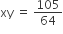
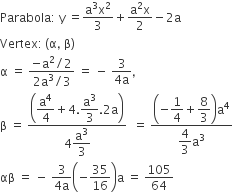
In an ellipse, the distance between its foci is 6 and minor axis is 8. Then its eccentricity is
3/5
1/5
2/5
2/5
If the lines 3x − 4y − 7 = 0 and 2x − 3y − 5 = 0 are two diameters of a circle of area 49π square units, the equation of the circle is
x2 + y2 + 2x − 2y − 47 = 0
x2 + y2 + 2x − 2y − 62 = 0
x2 + y2 − 2x + 2y − 62 = 0
x2 + y2 − 2x + 2y − 62 = 0
Let C be the circle with centre (0, 0) and radius 3 units. The equation of the locus of the mid points of the chords of the circle C that subtend an angle of 2π/3 at its centre is
x2+y2 = 3/2
x2 + y2 = 1
x2+y2 = 27/4
x2+y2 = 27/4
Let P be the point (1, 0) and Q a point on the locus y2 = 8x. The locus of mid point of PQ is
y2 – 4x + 2 = 0
y2 + 4x + 2 = 0
x2 + 4y + 2 =
x2 + 4y + 2 =
The locus of a point P (α, β) moving under the condition that the line y = αx + β is a tangent to the hyperbola 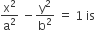
an ellipse
a circle
a parabola
a parabola
