 Multiple Choice Questions
Multiple Choice QuestionsIf is the area of the triangle formed by the positive x-axis and the normal and tangent to the circle x2 + y2 = 4 at (1, ) then is equal to
6
 Match The Following
Match The FollowingGiven the circle C with the equation x2 + y2 - 2x + 10y - 38 = 0. Match the List I with the List II given below concerning C
| List I | List II | ||
| A | The equation of the polar of (4, 3)with respect to C | I | y + 5 = 0 |
| B | The equation of the tangent at (9, - 5) on C | II | x = 1 |
| C | The equation of the normal at(- 7, - 5) on C | III | 3x + 8y = 27 |
| D | The equation of the diameter of C passing through (1,3) | IV | x + y = 3 |
| V | x = 9 |
The correct answer is
| A. A B C D | (i) III I V II |
| B. A B C D | (ii) IV V I II |
| C. A B C D | (iii) III V I II |
| D. A B C D | (iv) IV II I V |
For the circle C with the equation x2 + y2 - 16 - 12y + 64 = 0 match the List I with the List II given below.
| List I | List II | ||
| (i) | The equation of the polar of (- 5, 1) with respect to | (A) | y = 0 |
| (ii) | The equation of the tangent at (8, 0) to C | (B) | y = 6 |
| (iii) | The equation of the normal at (2, 6) to C | (C) | 13x + 5y = 98 |
| (iv) | The equation of the diameter of C through (8, 12) | (D) | 13x + 5y = 98 |
| (E) | x = 6 |
The correct match is
| A. (i) (ii) (iii) (iv) | (i) (D) (B) (A) (E) |
| B. (i) (ii) (iii) (iv) | (ii) (D) (A) (B) (E) |
| C. (i) (ii) (iii) (iv) | (iii) (C) (D) (A) (B) |
| D. (i) (ii) (iii) (iv) | (iv) (C) (E) (B) (A) |
 Multiple Choice Questions
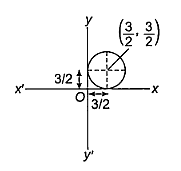
Multiple Choice QuestionsThe circle
Touches both the axes
Touches the x-axis only
Touches the y-axis only
Does not touch the axes
A.
Touches both the axes
If the length of the tangent from (h, k) to the circle x2 + y2 = 16 is twice the length of the tangent from the same point to the circle x2 + y2 + 2x + 2y = 0, then
(, 0) and (b, 0) are centres of two circles belonging to a coaxial system of which y-axis is the radical axis. If radius of one of the circles is 'r', then the radius of the other circle is
If the circle x2 + y2 + 4x - 6y + c =0 bisects the circumference of the circle x2 + y2 - 6x + 4y - 12 = 0, then c is equal to
16
24
- 42
- 62
A circle of radius 4, drawn on a chord of the parabola y2 = 8x as diameter, touches the axis of the parabola. Then, the slope of the chord is
1
2
The mid-point of a chord of the ellipse x2 + 4y2 - 2x + 20y = 0 is (2, - 4). The equation of the chord is
x - 6y = 26
x + 6y = 26
6x - y = 26
6x + y = 26
