If (1, 1, 1), (1, - 1, 1), (- 7, - 3, - 5) and (p, 2, 3) are coplanar, then the value of p will be

• 5

• 3

• 2

• None of these

If u, v, ware three non-coplanar vectors, then (u + v - w){(u - v) x (v - w)} is equal to

• $\mathrm{u} . \left(\mathrm{v} \times \mathrm{w}\right)$

• $\mathrm{v} . \left(\mathrm{u} \times \mathrm{w}\right)$

• $\mathrm{w} . \left(\mathrm{u} \times \mathrm{v}\right)$

• 0

If the vectros  $\hat{\mathrm{i}} - 3\hat{\mathrm{j}} + 2\hat{\mathrm{k}}$, $- \hat{\mathrm{i}} + 2\hat{\mathrm{j}}$ represents the diagonals ofa parallelogram, then its area will be

• $\sqrt{21} \mathrm{sq} \mathrm{unit}$

• $\frac{\sqrt{21}}{2} \mathrm{sq} \mathrm{unit}$

• $2\sqrt{21} \mathrm{sq} \mathrm{unit}$

• $\frac{\sqrt{21}}{4} \mathrm{sq} \mathrm{unit}$

334.

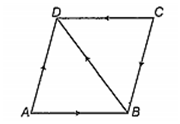
If ABCD is a parallelogram. AB = $2\hat{\mathrm{i}} + 4\hat{\mathrm{j}} - 5\hat{\mathrm{k}}$ and AD = $\hat{\mathrm{i}} + 2\hat{\mathrm{j}} + 3\hat{\mathrm{k}}$, then unit vector in the direction of BD is

• $\frac{1}{\sqrt{69}}\left(\hat{\mathrm{i}} + 2\hat{\mathrm{j}} - 8\hat{\mathrm{k}}\right)$

• $\frac{1}{69}\left(\hat{\mathrm{i}} + 2\hat{\mathrm{j}} - 8\hat{\mathrm{k}}\right)$

• $\frac{1}{\sqrt{69}}\left(- \hat{\mathrm{i}} - 2\hat{\mathrm{j}} + 8\hat{\mathrm{k}}\right)$

• $\frac{1}{69}\left(- \hat{\mathrm{i}} - 2\hat{\mathrm{j}} + 8\hat{\mathrm{k}}\right)$

The coordinates of foot of perpendicular drawn from the origin on the line formed by joining the points (- 9, 4, 5)and (10, 0, - 1), are

• (- 3, 2, 1)

• (1, 2, 2)

• (4, 5, 3)

• None of these

If the direction cosines of two lines are represented by l + m + n = 0 and 2lm + 2nl - mn = 0, then the angle between these lines will be

• $\frac{\mathrm{\pi }}{3}$

• $\frac{2\mathrm{\pi }}{3}$

• $\mathrm{\pi }$

• None of these

The direction ratios of the diagonals of a cube which joins the origin to the opposite corner are (when the 3 concurrent edges of the cube are coordinate axes)

• $\frac{2}{\sqrt{3}}, \frac{{\displaystyle 2}}{{\displaystyle \sqrt{3}}}, \frac{{\displaystyle 2}}{{\displaystyle \sqrt{3}}}$

• 1, 1, 1

• 2, - 2, 1

• 1, 2, 3

The equation of the plane in which the lines $\frac{\mathrm{x} - 5}{4} = \frac{\mathrm{y} - 7}{4} = \frac{\mathrm{z} + 3}{- 5} \mathrm{and} \frac{\mathrm{x} - 8}{7} = \frac{\mathrm{y} - 4}{1} = \frac{\mathrm{z} - 5}{3}$ lie, is

• 17x - 47y - 24z + 172 = 0

• 17x + 47y - 24z + 172 = 0

• 17x + 47y + 24z + 172 = 0

• 17x - 47y + 24z + 172 = 0

If the lines $\frac{\mathrm{x} - 1}{- 3} = \frac{\mathrm{y} - 2}{2\mathrm{k}} = \frac{\mathrm{z} - 3}{2}$ and $\frac{\mathrm{x} - 1}{3\mathrm{k}} = \frac{\mathrm{y} - 5}{1} = \frac{\mathrm{z} - 6}{- 5}$ are at right angles, then the value of k will be

• $- \frac{10}{7}$

• $- \frac{7}{10}$

• - 10

• 7

The coordinates of the point where the line $\frac{\mathrm{x} - 6}{- 1} = \frac{\mathrm{y} + 1}{0} = \frac{\mathrm{z} + 3}{4}$ meets the plane x + y - z = 3, are

• (2, 1, 0)

• (7, - 1, 7)

• (1, 2, - 6)

• (5, - 1, 1)