Previous Year Papers

Download Solved Question Papers Free for Offline Practice and view Solutions Online.

Test Series

Take Zigya Full and Sectional Test Series. Time it out for real assessment and get your results instantly.

Test Yourself

Practice and master your preparation for a specific topic or chapter. Check you scores at the end of the test.

Oscillations

Multiple Choice Questions

191.
Which of the following functions represents a simple harmonic oscillation?

sinωt $-$ cosωt

sin²ωt

sinωt + sin2ωt

sinωt $-$ sin2ωt

sinωt $-$ cosωt

(a) SHM

The given function is

sin ωt $-$ cos ωt

Multiply and divide by $\sqrt{2}$ on both side

⇒ = $\sqrt{2} [\frac{1}{\sqrt{2}} sinωt - \frac{1}{\sqrt{2}} cosωt]$

= $\sqrt{2} [sinωt \times \cos \frac{π}{4} - cosωt \times \sin \frac{π}{4}]$

Since $\cos \frac{π}{4} = \frac{1}{\sqrt{2}} and \sin \frac{π}{4} = \frac{1}{\sqrt{2}}$

= $\sqrt{2} \sin (ωt - \frac{π}{4})$

The function represent the SHM as it can be written in the form

$α = \sin (ωt + ϕ)$

Its period is $\frac{2 π}{ω}$

(b) Periodic but not SHM

the wave sin²ωt is periodic but not SHM because the acceleration in this motion is not proportional to the displacement yet it is periodic.

sin²ωt = $\frac{1 - \cos 2 ωt}{2}$

⇒ sinωt = $\sqrt{\frac{1 - \cos 2 ωt}{2}}$

When t = T then 1 $-$ cos2ωt = 0

cos2ωt = 1

2ωt = 2 $π$

⇒ T = $\frac{π}{ω}$

Hence the option (a) represents the periodic function.

192.

A magnet is made to oscillate with a particular frequency, passing through a coil as shown in figure. The time variation of the magnitude of e.m.f. generated across the coil during one cycle is

193.

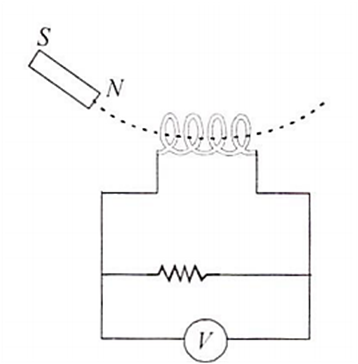
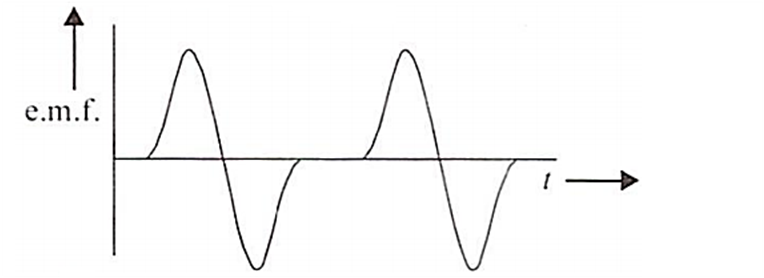
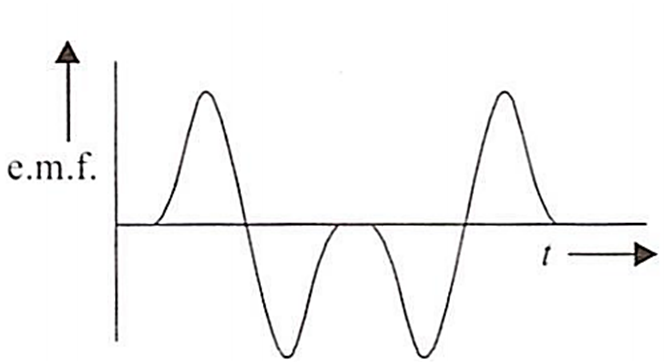
Assertion: The amplitude of an oscillating pendulum decreases gradually with time.

Reason: The frequency of the pendulum decreases with time.

if both assertion and reason are true and reason is the correct explanation of assertion
if both assertion and reason are true and the reason is not the correct explanation of the assertion
if assertion is true but reason is false
if both assertion and reason are false statements

194.

A string in a musical instrument is 50 cm long and its fundamental frequency is 800 Hz. If a frequency of 1000 Hz is to be produced, then required length of string is

62.5 cm
40 cm
50 cm
37.5 cm

195.

Assertion: The time-period of pendulum, on a satellite orbiting the earth is infinity.

Reason: Time-period of a pendulum is inversely proportional to $\sqrt{g}$

If both the assertion and reason are true and reason is a correct explanation of the assertion.
If both assertion and reason are true but assertion is not a correct explanation of the assertion.
If the assertion is true but the reason is false.
If both assertion and reason are false.

196.

Assertion: In simple harmonic motion, the motion is to and fro and periodic.

Reason: Velocity of the particle ( v) = ω $\sqrt{k^{2} - x^{2}}$ ( where x is the displacement ).

If both the assertion and reason are true and reason is a correct explanation of the assertion.
If both assertion and reason are true but assertion is not a correct explanation of the assertion.
If the assertion is true but the reason is false.
If both assertion and reason are false.

197.

The time period of a simple pendulum is T remaining at rest inside a lift. Find the time period of pendulum when lift starts to move up with an acceleration of g/4

T
T/2
$\frac{2 T}{5}$
$\frac{2 T}{\sqrt{5}}$

198.

From the given figure find the frequency of oscillation of the mass m.

199.

A simple pendulum is made by attaching a 1 kg bob to a 5 m long copper wire. Its period is T. Now, if 1 kg bob is replaced by 10 kg bob, the period of oscillations