 Multiple Choice Questions
Multiple Choice QuestionsA body of mass m is travelling with a velocity u. When a constant retarding force F is applied, it comes to rest after travelling a distances s1. If the initial velocity is 2u, with the same force F, the distance travelled before it comes to rest is s2. Then,
s2 = 4s1
s2 = 2s1
s2 = s1
A block kept on a rough surface starts sliding when the inclination of the surface is with respect to the horizontal. The coefficient of static friction between the block and the surface is
sec θ
sin θ
tan θ
cos θ
Two bodies ofmasses m1 and m2 are acted upon by a constant force F for a time t. They start from rest and acquire kinetic energies, E1 and E2 respectively. Then is
1
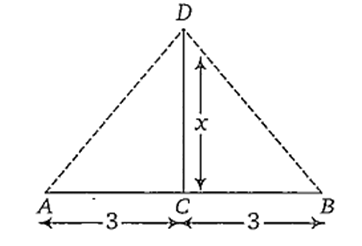
Two fixed charges A and B of 5 µC each are separated by a distance of 6 m. C is the mid point of the line joining A and B. A charge Q of −5μC is shot perpendicular to the line joining A and B through C with a kinetic energy of 0.06 J. The charge Q comes to rest at a point D. The distance CD is
4 m
3 m
A.
4 m
By conservation of energy
Loss of PE = Gain in KE
The dimensional formula of physical quantity is is [MaLbTc]. Then, that physical quantity is
Spring constant if a = 1, b = −1, c = −2
surface tension if a= 1, b = 1,c = −2
force if a = 1, b = 1, c = 2
angular frequency if a = 0, b = 0, c = − 1
A person throws balls into air vertically upward in regular intervals of time of one second. The next ball is thrown when the velocity of the ball thrown earlier becomes zero. The height to which the balls rise is (Assume, g = 10 ms-2)
20 m
5 m
10 m
7.5 m
A planet moving around sun sweeps area A1 in 2 days, A2 in 3 days and A3 in 6 days. Then, the relation between A1, A2 and A3 is

6A1 = 3A2 = 2A3
3A1 = 2A2 = A3
2A1 = 3A2 = 6A3
3A1 = 2A2 = 6A3
