Subject

Mathematics

Class

ICSE Class 10

Pre Boards

Practice to excel and get familiar with the paper pattern and the type of questions. Check you answers with answer keys provided.

Sample Papers

Download the PDF Sample Papers Free for off line practice and view the Solutions online.

ICSE Class 10 Mathematics Solved Question Paper 2012

Short Answer Type

If A = $[\begin{matrix} 3 & 1 \\ - 1 & 2 \end{matrix}]$ and B = $[\begin{matrix} 1 & 0 \\ 0 & 1 \end{matrix}]$ , Find A² - 5A + 7I

The monthly pocket money of Ravi and Sanjeev are in the ratio 5:7. Their expenditures are in the ratio 3:5. If each saves Rs. 80 every month, find their monthly pocket money

3.
Using the Remainder Theorem factorise completely the following polynomial.
3x³ + 2x² – 19x + 6

Given equation is, 3x³ + 2x²- 19x + 6

Let p(x) = 3x³ + 2x² – 19x + 6

By hit and trial method,

P(–1) = 3(–1)³ + 2(–1)² – 19(–1) + 6 = –3 + 2 + 19 + 6 = 24 ≠ 0

P(1) = 3(1)³ + 2(1)² – 19(1) + 6 = 3 + 2 – 19 + 6 = –8 ≠ 0

p(2) = 3(2)³ + 2(2)² – 19(2) + 6 = 24 + 8 – 38 + 6 = 0

Thus, (x – 2) is a factor of p(x).

Now,

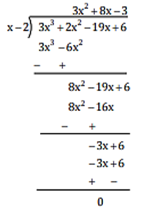
Therefore, 3x³ + 2x² – 19x + 6 = (x - 2)(3x² + 8x - 3)

= (x - 2)(3x² + 9x - x - 3)

= (x - 2)[3x(x + 3) - 1(x + 3)]

= (x - 2)(x + 3)(3x - 1)

Thus, x = 2, -3, 1/3

On what sum of money will the difference between the compound interest and simple interest for 2 years be equal to Rs. 25 if the rate of interest charged for both is 5% p.a.?

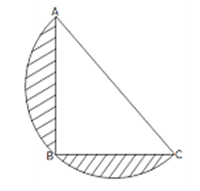
ABC is an isosceles right angled triangle with ∠ABC = 90 $°$ . A semi-circle is drawn with AC as the diameter. If AB = BC = 7 cm, find the area of the shaded region.
Take ( $π = \frac{22}{7}$ )

Given a line segment AB joining the points A(– 4, 6) and B(8, – 3). Find

(i) The ratio in which AB is divided by y-axis.

(ii) Find the coordinates of the point of intersection.

(iii) The length of AB.

In the given figure O is the centre of the circle and AB is a tangent at B. If AB = 15 cm and AC = 7.5 cm. Calculate the radius of circle.

Evaluate without using trigonometric tables:

$\cos^{2} (26 °) + \cos (64 °) \sin (26 °) + \frac{\tan (36 °)}{\cot (54 °)}$

Marks obtained by 40 students in a short assessment is given below, where a and b are two missing data.

Marks 5 6 7 8 9

Number of Students 6 a 16 13 b

If the mean of the distribution is 7.2, find a and b.

10.

Kiran deposited Rs. 200 per month for 36 months in a bank’s recurring deposit account. If the bank pays interest at the rate of 11% per annum, find the amount she gets on maturity.