 Multiple Choice Questions
Multiple Choice QuestionsThe area between the parabola y = x2 and the line y = x is :
None of these
A.
Equation of parabola is
y = x2 ...(i)
Equation of straight line is
y = x ...(ii)
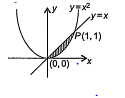
FromEqs. (i) and (ii)
x2 - x = 0 x(x - 1) = 0
x = 0, 1 y = 0, 1
Hence, point of intersections are (0, 0) and (1, 1).
The solution of the differential equation sec2(x)tan(y))dx + sec2(y)tan(x))dy = 0 is :
None of these
are three vectors with magnitude and such that is perpendicular to , is perpendicular to is perpendicular to . It follows that is equal to :
9
6
5
4
