 Multiple Choice Questions
Multiple Choice QuestionsThe area of the plane region bounded by the curve x = y2 - 2 and the· line y = - x is (in square units)
C.
Given curves x = y2 - 2 and y = x
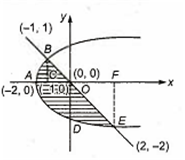
Thus, interection point are
(- 1, 1) and (2, - 2)
We are to find the area of shaded part
Area of ABC =
=
Area of BCO =
Area of ADO
Area of ODE = area of ODEF - area of OPE
[ neglecting the negative sign]
=
=
=
= sq unit
The family of curves y = easin(x), where a is anarbitrary constant, is represented by thedifferential equation
The solution of the differential equation is
x + ex + y = c
x - ex + y = c
x + e- (x + y) = c
x - e- (x + y) = c
The degree and order of the differential equation where p = , are respectively.
3, 1
1, 3
1, 1
3, 3
If the distance between (2, 3) and (- 5, 2) is equal to the distance between (x, 2) and (1, 3), then the values of x are
- 6, 8
6, 8
- 8, 6
- 7, 7
