Show that ΔABC, where A(–2, 0), B(2, 0), C(0, 2) and ΔPQR where P(–4, 0), Q(4, 0), R(0, 2) are similar triangles.

In ΔABC, the coordinates of the vertices are A(–2, 0), B(2, 0), C(0, 2).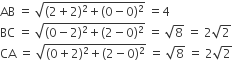

$AB space equals space square root of left parenthesis 2 plus 2 right parenthesis squared plus left parenthesis 0 minus 0 right parenthesis squared end root space equals 4 BC space equals space square root of left parenthesis 0 minus 2 right parenthesis squared plus left parenthesis 2 minus 0 right parenthesis squared end root space equals space square root of 8 space equals space 2 square root of 2 CA space equals space square root of left parenthesis 0 plus 2 right parenthesis squared plus left parenthesis 2 minus 0 right parenthesis squared end root space equals space square root of 8 space equals space 2 square root of 2$

In ΔPQR, the coordinates of the vertices are P(–4, 0), Q(4, 0), R(0, 4).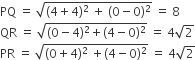
$PQ space equals space square root of left parenthesis 4 plus 4 right parenthesis squared space plus space left parenthesis 0 minus 0 right parenthesis squared end root space equals space 8 QR space equals space square root of left parenthesis 0 minus 4 right parenthesis squared plus left parenthesis 4 minus 0 right parenthesis squared end root space equals space 4 square root of 2 PR space equals space square root of left parenthesis 0 plus 4 right parenthesis squared space plus left parenthesis 4 minus 0 right parenthesis squared end root space equals space 4 square root of 2$

Now, for ΔABC and ΔPQR to be similar, the corresponding sides should be proportional.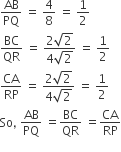
$AB over PQ space equals space 4 over 8 space equals space 1 half fraction numerator begin display style BC end style over denominator begin display style QR end style end fraction space equals space fraction numerator begin display style 2 square root of 2 end style over denominator begin display style 4 square root of 2 end style end fraction space equals space fraction numerator begin display style 1 end style over denominator begin display style 2 end style end fraction fraction numerator begin display style CA end style over denominator begin display style RP end style end fraction space equals space fraction numerator begin display style 2 square root of 2 end style over denominator begin display style 4 square root of 2 end style end fraction space equals space fraction numerator begin display style 1 end style over denominator begin display style 2 end style end fraction So comma space fraction numerator begin display style AB end style over denominator begin display style PQ end style end fraction space equals fraction numerator begin display style BC end style over denominator begin display style QR end style end fraction space equals fraction numerator begin display style CA end style over denominator begin display style RP end style end fraction$
Thus, ΔABC is similar to ΔPQR.

2835 Views

Triangles

Hope you found this question and answer to be good. Find many more questions on Triangles with answers for your assignments and practice.

Mathematics

Browse through more topics from Mathematics for questions and snapshot.