
We are to maximise
Z = 3x + 4 y
subject to the constraints
x + y ≤ 4
x ≥ 0, y ≥ 0
Consider a set of rectangular cartesian axes OXY in the plane.
It is clear that any point which satisfies x ≥ 0, y ≥ 0 lies in the first quadrant.
Let us draw the graph of x + y = 4
For x = 0, y = 4
For y = 0, x = 4
∴ line meets OX in A(4, 0) and OY in L(0, 4)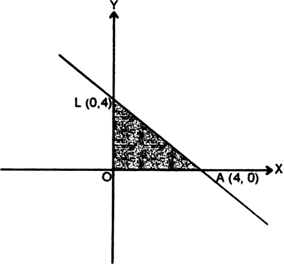
Since feasible region is the region which satisfies all the constraints.
∴ OAL is the feasible region. The comer points are O(0, 0), A(4, 0), L(0, 4).
At O(0, 0), Z = 0 + 0 = 0
At A(4, 0), Z = 12 + 0 = 12
At L(0, 4), Z = 0 + 16 = 16
∴ maximum value = 16 at (0, 4).
We are to minimise
Z = - 3x + 4 y
subject to the constraints
x + 2 y ≤ 8
3x + 2 y ≤ 12
x ≥ 0, y ≥ 0
Consider a set of rectangular cartesian axes OXY in the plane.
It is clear that any point which satisfies x ≥ 0, y ≥ 0 lies in the first quadrant.
Let us draw the graph of x + 2 y = 8
For x = 0, 2 y = 8 or y = 4
For y = 0, x = 8
∴ line meets OX in A(8, 0) and OY in L(0, 4).
Again we draw the graph of 3x + 2 y = 12
For x = 0, 2 y = 12 or y = 6
For y = 0, 3x = 12 or x = 4
∴ line meets OX in B(4, 0) and OY in M(0, 6).
Since feasible region is the region which satisfies all the constraints.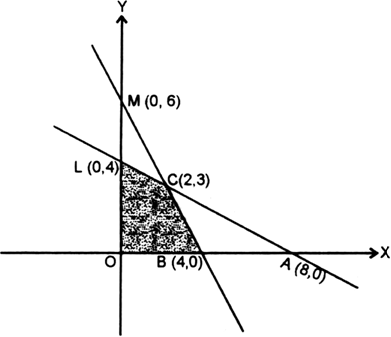
∴ OBCL is the feasible region and O(0, 0), B(4, 0), C(2, 3), L(0, 4) are comer points.
At O(0, 0), Z = 0 + 0 = 0
At B(4, 0), Z = -12 + 0 = -12
At C(2, 3), Z = -6 + 12 = 6
At L(0, 4), Z = 0 + 16 = 16
∴ minimum value = -12 at (4, 0).
We have to maximise
Z = 5x + 3 y
subject to the constraints
3x + 5 y ≤ 15
5x + 2 y ≤ 10
x ≥ 0, y ≥ 0
Consider a set of rectangular cartesian axes OXY in the plane.
It is clear that any point which satisfies x ≥ 0, y ≥ 0 lies in the first quadrant.
Let us draw the graph of 3x + 5 y= 15
For x = 0, 5 y = 15 or y = 3
For y = 0, 3x = 15 or x = 5
∴ line meets OX in A(5, 0) and OY in L(0, 3).
Again we draw the graph of 5x + 2 y = 10
For x = 0, 2 y = 10 or y = 5
For y = 0, 5x = 10 or x = 2
∴ line meets OX in B(2, 0) and OY in M(0, 5).
Since feasible region is the region which satisfies all the constraints.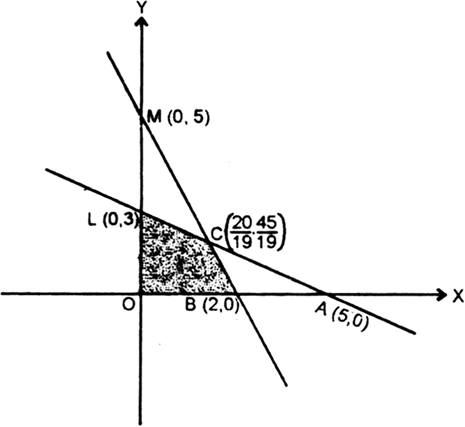
∴ OBCL is the feasible region and corner points are O(0, 0), B(2, 0),
![]()
At ![]()
![]()
At ![]()
![]()
At ![]()
![]()
![]()
We are to minimise
Z = 3x + 2y
subject to the constraints
x + 2y ≤ 10
3x + y ≤ 15
x, y ≥ 0
Consider a set of rectangular cartesian axes OXY in the plane.
It is clear that any point which satisfies x ≥ 0, y ≥ 0 lies in the first quadrant.
Let us draw the graph of x + 2y = 10.
For x = 0, 2y = 10 or y = 5
For y = 0, x = 10
∴ line meets OX in A(10, 0) and OY in L(0, 5).
Again we draw the graph of 3x + y = 15.
For x = 0, y = 15
For y = 0, 3 x = 15 or x = 5
∴ line meets OX in B(5, 0) and OY in M(0, 15).
Since feasible region is the region which satisfies all the constraints.
∴ OBCL is the feasible region.
The comer points O(0, 0), B(5, 0), C(4, 3), L(0, 5).
At O(0, 0), Z = 0 + 0 = 0
At B(5, 0), Z = 15 + 0 = 15
At C(4, 3), Z = 12 + 6 = 18
At L(0, 5), Z = 0 + 10 = 10
∴ maximum value = 18 at (4, 3).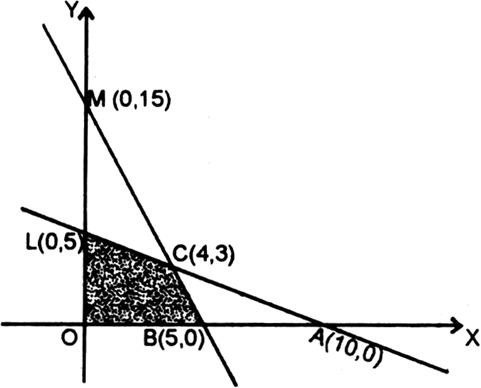
We are to minimise and maximise Z = 5x + 10y subject to constraints x + 2 y ≤ 120
x + y ≥ 60
x - 2y ≥ 0
x, y ≥ 0
Consider a set of rectangular cartesian axes OXY in the plane.
It is clear that any point which satisfies x ≥ 0, y ≥ 0 lies in the first quadrant.
Let us draw the graph of x + 2 y = 120
For x = 0, 2 y = 120 or y = 60
For y = 0, x = 120
∴ line meets OX in A( 120, 0) and OY in L(0, 60).
Also we draw the graph of
x + y = 60.
For r = 0, y = 60
For y = 0, x = 60
∴ line meets OX in B(60, 0) and OY in L(0, 60).
Again we draw the graph of
x - 2y = 0
This is a line through the origin and C(40, 0), which is point of intersection of x - 2 y = 0 and x + y = 60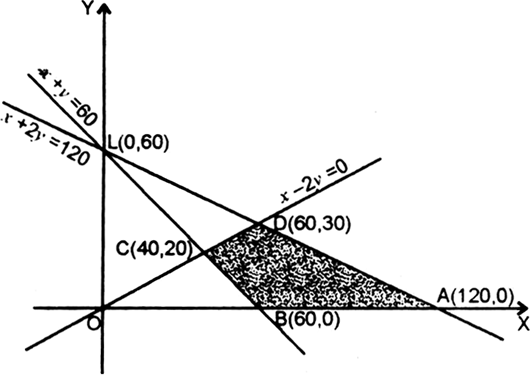
Since feasible region satisfies all the constraints.
∴ BADC is the feasible region.
Comer points are B(60, 0), A(120, 0), D(60, 30), C(40, 20).
At B(60, 0), Z = 300 + 0 = 300
At A(120, 0), Z = 600+ 0 = 600
At D(60, 30), Z = 300 + 300 = 600
At C(40, 20), Z = 200 + 200 = 400
∴ minimum value = 300 at (60, 0) and maximum value = 600 at (120, 0) and (60, 30).
