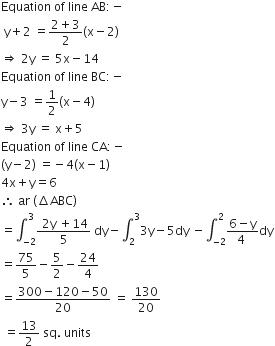The shaded area OBAO represents the area bounded by the curve x² = 4y and the line x = 4y – 2.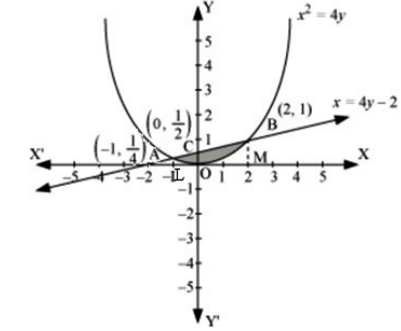


Let A and B be the points of intersection of the line and parabola.
Co-ordinates of point A are 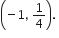 Co-ordinates of point B are (2, 1).
Area OBAO = Area OBCO + Area OACO   ...(1)
Area OBCO = 

Area OACO = 
  
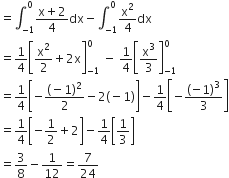
Therefore, required area = 

Given equations of the circles are:

Equation (1) is a circle with centre O at the origin and radius 2. Equation (2) is a circle with centre C(2, 0) and radius 2.
Solving (1) and (2), we have:

This gives 
Thus, the points of intersection of the given circles are 

Required area
 = Area of the region OACA'O
 = 2[area of the region ODCAO] 
 =2[area of the region ODAO + area of the region DCAD]

Consider the given equation. 

This equation represents a semicircle with centre at the origin and radius  = 
Given that the region is bounded by the above semicircle and the line 
Let us find the point of intersection of the given curve meets the line 

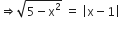
Squaring both the sides, we have,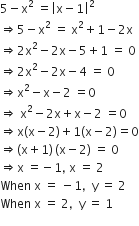


Consider the following figure
Thus the intersection points are 1,2 and 2,1 ( ) ( ) Consider the following sketch of the bounded region.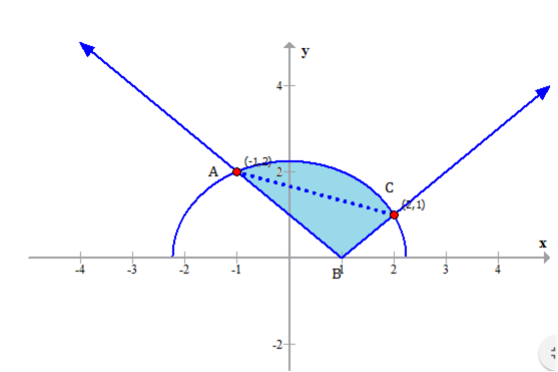


Required Area, 

Consider the vertices, A(-1, 2), B(1, 5) and C(3, 4).
Let us find the equation of the sides of the triangle 
Thus, the equation of AB is:




Now the area of  = Area of  + Area of