The acceleration due to gravity changes with the angle of latitude due to shape and rotation of the earth about its own axis.
Variation of g due to shape:
The earth is not a prefect sphere. It is flat at the pole and bulges out at the equator as a result polar radius is smaller than equatorial radius. As the acceleration due to gravity is inversely proportional to the square of radius of the earth, therefore the value of g increases as one moves from equator to pole.
Variation of g due to rotation:
We know that the earth rotates about its own axis as a result of which the objects on the earth experience the centrifugal force. This centrifugal force decreases the acceleration due to gravity and magnitude of change in the value of g due to rotation of the earth depends on the value of the angle of latitude.
Let us consider the earth to be a homogenous sphere of mass Mand radius R. The earth rotates about polar axis and let ω be the angular velocity of rotation of the earth. All the objects at rest on earth also revolve about its polar axis with same angular velocity ω. Let us consider a body of mass m placed at a point P on the earth at angle of latitude λ.
If the earth were at rest, then the body would have been attracted towards the center the center of the earth with force mg.But due to rotation of earth, the body describes a circle of radius OP = r = ![]() with center at O. In rotating frame the earth, body experiences pseudo force
with center at O. In rotating frame the earth, body experiences pseudo force ![]() along
along ![]() Now the apparent weight of the body is resultant of force mg directed towards the center and force
Now the apparent weight of the body is resultant of force mg directed towards the center and force ![]() along
along ![]()
Applying parallelogram of vector addition, we get![]()
or ![]()
![]()
As ![]() therefore
therefore ![]() can be neglected.
can be neglected.
∴ ![]()
![]() [using binomial exp]
[using binomial exp]
or ![]()
Substituting ![]() we get
we get
![]()
The weight of a body at depth d is equal to the weight of the body at height h above the surface of the earth. What is the ratio of h to d, for small value of h and d?
OR
Prove that the distance we have to cover into the earth below its surface is twice the distance we have to cover above the surface of the earth to get the same change in the weight of a body?
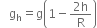

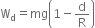


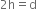


We know that acceleration due to gravity at equator is,

where,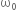 is the angular velocity of the earth.
is the angular velocity of the earth.
Angular velocity of Earth= 
If the acceleration due to gravity at the equator becomes zero, the body at equator experiences weightlessness.
Let  be the angular velocity of rotation at which the acceleration due to gravity at the equator becomes zero.
be the angular velocity of rotation at which the acceleration due to gravity at the equator becomes zero.
That is,
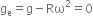

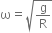
Now,



Thus, the earth should rotate 17 times faster than the present rate.
Rotation speed increases by a factor of 17.
Therefore, the duration of day decreases by factor 17.
New duration of day,

According to Newton’s law of gravitation, every mass exerts gravitational force on every other mass. Thus the earth exerts force on every mass placed surrounding it. This force of attraction exerted by the earth on a body is called force of gravity and the acceleration possessed by a body moving under the force of gravity is called acceleration due to gravity.
The value of acceleration due to gravity does not depend on the mass of a body but it depends on the mass of the earth and distance of the body from the center of the earth.
Let us consider the earth to be a uniform sphere of mass M and radius R. Let a body be placed at a distance r from the surface of the earth. The force of gravity on the body is,![]()
The acceleration produced in the body due to force of gravity i.e. acceleration due to gravity is,![]()
At the surface of the earth, the acceleration due to gravity is,![]()

